Introduction
Nearly all present-day physicists are convinced of the truth of the assertion in the following quotation from Weidner and Sells’ Elementary Modern Physics(1):
It was by the alphaparticle scattering experiments, suggested by Rutherford, that the existence of atomic nuclei was established.
However, when we study the literature of Rutherford’s era, we find that he and his associates, Geiger and Marsden, did not in fact discover the atomic nucleus. Geiger and Marsden’s paper, ”The Laws of Deflexion of a Particles through Large Angles, ”(2) does present strong experimental evidence of a central repulsive force originating from atoms, but the paper does not prove that this force is electrical in nature. What their experiments did prove is that the number of particles scattered through an angle q is proportional to 1/sin4 (q/2) and to the inverse of the square of the kinetic energy of the particles, 1/Ek2. Of course, the experiments did disprove the Thomson ”plum pudding ” atom model, which did not predict a strong central repulsive force; but it is one thing to disprove a theory; it is quite another to prove one. If the Rutherford model were the only alternative left, we might have to conclude that it is correctÑbut there are always other alternatives. This paper will present one such alternative: the Reciprocal System of physical theory. A new scattering equation will be derived and compared with the experimental facts as found in an uptodate version of the original experiment, that conducted by Prof. Adrian C. Melissinos and his students.(3) The originator of the Reciprocal System is Dewey B. Larson; for full comprehension of this paper, the reader should first study Larson’s books.(4,5,6)
I. Theory
A. The Repulsion Force: F
1. The Reciprocal System
In the Reciprocal System, nonionized and nonmagnetized matter is subject to only two primary forces: the spacetime progression and gravitation. In the timespace region, the progression is outward and gravitation inward, whereas in the time region (inside unit space) the progression is inward and gravitation outward -- a repulsion. Right at the boundary the progression is zero, but the net gravitation is not zero. Compared with the repulsive gravitational force, the attractive gravitational force is negligible. Thus at the boundary only the repulsive gravitational force is effective. Now consider what happens when an atom A, which is moving towards an atom B, reaches this boundary. According to Larson,(6)
When atom A reaches point X, one unit of space distant from B, it cannot move any closer to B in space. It is, however, free to change its position in time relative to the time location occupied by atom B. The reciprocal relation between space and time makes an increase in time separation equivalent to a decrease in space separation, and while atom A cannot move closer to atom B in space, it can move to the equivalent of a spatial position that is closer to B by moving outward in coordinate time. . . . No matter what the spatial direction of the motion of the atom may have been before unit distance was reached, the temporal direction of the motion after it makes the transition to motion in time is determined purely by chance.
A previous paper of mine, ”Time Region Particle Dynamics, ”(7) dealt with the situation in which atom A (say an alpha particle) is assumed to continue to move directly toward atom B (say a gold atom) in the time region. This paper will consider the general case in which no assumption is made as to the actual motion that takes place in the equivalent space of the time region. All that will be considered here is the equivalent motion that takes place at the boundary, i.e., in actual space. Here, with the atoms separated by so, the repulsive gravitational force F is (from Ref. 4)
| F = KG/s4 = KG/so4 (if s = so) |
(1)
|
The repulsion coefficient KG is expressed by
| KG = [Fpso4/(156.44)4] * [ln4 teff/ln²t’eff] |
(2)
|
where Fp is the natural unit of force and the number 156.44 is the interregional ratio. The dimensionless variables teff and t’eff are material constants determined by the characteristics of the interacting particles, and will be discussed further later.
2. Conventional Theory
In present theory, the alpha particles somehow avoid interacting with the cloud of electrons supposedly surrounding each gold nucleus. The only force involved comes from the presumed nucleus. At low energies this is a Coulombic force, given by
| F = zZe²/4peos² |
(3)
|
where z is the atomic number of the alpha particule (helium), Z is the atomic number of gold, e is the value of electric charge, eo is the permittivity constant of free space, and s is the separation distance. This is an inverse square force, rather than an inverse quartic force as in the Reciprocal System. Why this Coulombic force should act between particles but not within nuclei is a question completely unanswered by current theory.
B. The Impact Parameter: b
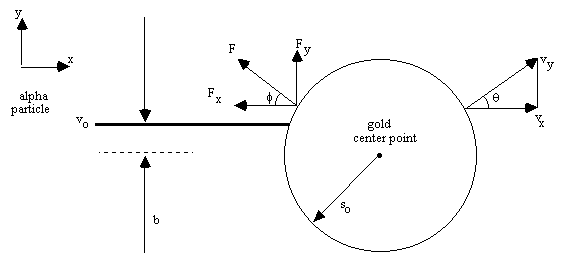
MOTION IN ACTUAL SPACE: so remains constant, but angle f changes from o to p-q where q is the deflection angle observed in the time-space region.
The figure shows a typical collision process. The impact parameter is the distance that the alpha particle would have passed the gold atom if there had been no force between them.
1. Reciprocal System
Let m be the mass of the alpha particle and vo be its initial velocity. Referring to the figure, we have
impulse = D(mv)y |
|
òFdt = mvo sin(q) |
|
(KG/so4) òsin(f)dt = mvosin(q) |
(4)
|
The alpha particle passes from the timespace region, through the time region, and back into the timespace region. For the general case, we cannot write an equation for the actual motion in the equivalent space of the time region, but we can write an equation for the equivalent motion in the actual space of the timespace region. Throughout this motion, neither the angular momentum, nor the actual spatial separation, changes. But the angle f does change. Therefore,
| mvob = mso²(df/dt) |
(5)
|
Separating dt from df in eq. (5) and substituting in eq. (4), we have
(KG/so4) * (so²/vob) òsin(f) d(f) = mvo sin(q) |
(6a) |
|
or |
(KG/so²mvo²b) òsin(f) d(f) = sin(q) |
Since the kinetic energy, Ek, is (1/2) mvo², eq. (6a) can be rewritten as
| [KG/(so² * 2 * Ek * b)] òsin(f) d(f) = sin(q) |
(6b)
|
Before the collision, f = 0 and after the collision, f = p-q, so these are the limits on the integral.
|
ò
|
p-q | / | p-q |
| sin(f) df = -cos(f) | |||
| o | o | ||
| = cos (p-q) + cos(o) | |||
| = cos(q) + 1 |
| Thus, | [KG/(so² * 2 * Ek * b)] * (cos(q) + 1) = sin(q) |
(7a)
|
| But | sin(q)/(cos(q) + 1) = tan (q/2) | |
| so | KG/(so2 * 2 * Ek * b) = tan(q/2) |
(7b)
|
Solving for b we finally obtain
| b = [KG/(2 * so² * Ek)] * cot(q/2) |
(7c)
|
2. Conventional Theory
Refs. 1, 3, and 8 all have derivations for b in current theory. The result is
| b = [zZe²/(8peoEk)] * cot (q/2) |
(8)
|
C. Target Cross-Section: s
The target Cross — Section is defined as s = pb².
1. The Reciprocal System
From eq. (7c), using the above, we obtain
| s = [pKG²/(4 * so4 * Ek²)] * cot² (q/2) |
(9)
|
2. Conventional Theory
With b from eq. (8),
| s = [z²Z²e4/(64 peo²Ek²)] * cot²(q/2) |
(10)
|
D. Differential Cross-Section: ds
The differential cross-section is
ds = 2 p bdb
1. Reciprocal System
| Here, | db = [-KG/(so² * 4 * Ek)] * [dq/sin²(q/2)] |
(11)
|
| Thus | ds = 2 p * [KG/(2 * so² * Ek)] * [cos(q/2)/sin(q/2)] | |
| * [-KG/(4 * so² * Ek)] * [dq/sin²(q/2)] | ||
| = [-2 p KG² cos(q/2) dq] / [8 * so4 * Ek² * sin³(q/2)] | ||
| = [p KG² cos(q/2) dq]/[4so4 * Ek² * sin³(q/2)] |
(12)
|
(with the minus sign dropped).
The angles q and q + dq define two cones with the horizontal line through the gold atoms as their axis. The differential solid angle dW between the two cones is
| dW = 2p sin(q) dq |
Since the cosine term-in eq. (12) can be expressed as
| cos(q/2) = sin(q)/[2 * sin(q/2)] |
eq. (12) becomes
| ds | = [pKG² (sin (q)) dq]/[8so4 * Ek² * sin4(q/2)] | ||
| = [KG² dW]/[16 * so4 * Ek² * sin4(q/2)] | |||
| or | ds/dW | = KG²/[16 * so4 * Ek² * sin4(q/2)] |
(13)
|
The units of ds/dW are meter squared per steradian, m²/sr.
2. Conventional Theory
Similarly, for conventional theory,
| ds/dW = z²Z²e4/[256 * p² * eo2 * Ek² * sin4(q/2)] |
(14)
|
E. The Scattering Constant: Ks
It is immediately seen from eqs. (13) and (14) that for both
theories,
| (ds/dW) * sin4(q/2) = Ks |
a constant.
1. Reciprocal System
Here,
| Ks = KG²/(16 * so4 * Ek²) |
(15)
|
2. Conventional Theory
Here,
| Ks = z²Z²e4/(256 * p²* eo² * Ek²) |
(16)
|
F. The Number of Particles Scattered per Minute at the angle q: Is
So far we have looked at the situation involving only one alpha particle and only one gold atom. For the situation in which a beam of alpha particles strikes a gold foil, we would like to compute the number of particles scattered through a certain angle q. Let
Io = number of incident alpha particles/minute
dW = detector solid angle
N = area density of scatterers (number of gold atoms/m2)
The value of N is determined from this equation:
| N = d * r * [No/A) * (104 cm²/m²)] | (17) |
where
d = thickness of foil (cm)
r = density of scatterer (g/cm3)
No = 6.02 * 1023, Avogadro’s number
A = atomic weight of scatterer
Then the number per minute, Is, of alpha particles scattered into the detector at the angle q is
|
Is
|
= Io * N (ds/dW) * dW |
| = Io * d * r * (No/A) * 104 * (ds/dW) * dW |
1. Reciprocal System
Here,
| Is = Io * d * r * (No/A) * 104 * KG²* dW/[16 * so4 * Ek2 * sin4(q/2)] |
(18)
|
2. Conventional Theory
Here,
| Is = Io * d * r * No/A * 104 * (z²Z²e4 * dW)/[256 * p² * eo² * Ek² * sin4(q/2)] |
(19)
|
Note: Both equations are based on the assumption that E is sufficiently low such that ”relativistic ” effects can be neglected and such that the gold atoms remain stationary during the interaction -- this would not be the case at very high alpha particle energies.
II. Experiment
A. The Experimental Set-up
Professor Adrian C. Melissinos carried out a modern version of the original GeigerMarsden experiment and described his findings in Ref. 3. In this experiment
| Io = 1.1 * 105 incident alpha particles/minute |
| dW D W = da/L2 = .8786/(6.67)² = 0.197 sr |
[da is differential area of detector and L is distance of detector from foil]
| r = 19.3 g/cm3 (gold) |
| d = .00025 cm |
| A = 197 (gold) |
Thus,
|
N
|
= d * r * (No/A) 104 |
| = (.00025)(19.3)(6.02 * 1023/197)*104 | |
|
N
|
= 1.4744 * 1023 gold atoms/m2 |
So the equation for Is is
|
Is
|
= Io * N * (ds/dW) * dW |
| = 1.1 * 105 * 1.4744 * 1023 * (ds/dW) * .0197 | |
|
Is
|
= 3.1950 * 1026 * ds/dW |
Is is measured, and then (ds/dW)is computed. For each angle q the product (ds/dW) * sin4(q/2) can be found and the results plotted. From leastsquares error analysis, the best fit experimental value of Ks can be obtained.
Melissinos states that the above value of Io, and hence also Is, is subject to at least a ± 20 percent error in view of the approximations used and the nonuniformities in beam density and direction. One other uncertainty is the energy of the alpha particles. The incoming energy is 5.2 MeV, but since the particles lose a considerable amount of energy in traversing the target, Melissinos believes that it is more appropriate to use a mean value of Ek for the calculations. He calculates the mean value to be
| Ek = 4.39 MeV = 7.03 * 10-13 J |
B. Calculation of Ks for the Experimental Set-up
1. Reciprocal System
Here,
| Ks = KG²/(16 * so4 * Ek²) |
| KG = [Fp * so4/(156.44)4] * [ln4 teff/ln² t’eff] |
| Fp = 3.27223 * 10-3 Newtons |
| so = 4.558816 * 10-8 meters |
| ln² t’eff = 1, since helium has no electric dispacement -- it is ”inert. ” |
The difficult part in calculating Keff for gold helium. The (tentative) method used here will be different from that used in my previous paper, Ref. 7. Gold has 3 active rotational dimensions with t = 4.5 in all (see Ref. 4 for more details). Helium has only 1 active dimension, with t = 3. The other two dimensions have t = 1. In the first dimension the mean value for gold and helium is
| t = (4.5 * 3)1/2 = 3.67 |
In the other two dimensions, the full rotational force of the gold atom is present, so instead of (4.5 * 1)1/2 = 2.1, we simply have t = 4.5. The mean over all three dimensions is
| teff = (3.67 * 4.5 * 4.5)1/3 = 4.2 |
Thus (tentatively),
|
KG
|
= [3.27223 * 103 * (4.558816 * 108)4/(156.44)4][ln4(4.2)] |
| = 1.00 * 1040 nm4 |
The (tentative) value of Ks is then Ks = (1.00 * 1040)2/[16 * (4.558816 * 108)4 * (7.03 * 1013)2] Ks = (2.93 * 1028 (m2/sr)
2. Conventional Theory
Here,
| Ks = z²Z²e4/(256 * p² * eo² * Ek²) |
| z = 2 |
| Z = 79 e = 1.602 * 1019 coulombs |
| eo = 8.85 * 1012 coulombs/N-m² |
| Ek = 7.03 * 1013 J |
Thus,
| Ks = [(2²)(79²)(1.602 * 1019)4] / [256 * p2 * (8.85 * 10-12)² * (7.03 * 10-13)²] Ks = 1.68 * 10-28 (m²/sr) |
3. Experiment
The best fit experimental value, according to Melissinos, is
| Ks = 2.70 * 1028 (m²/sr) |
Thus the theoretical and experimental results can be summarized as follows:
| Ks(m²/sr) | |
| Experiment: | 2.70*10-28 |
| Reciprocal Systems | 2.93*10-28 (tentatively) |
| Conventional Theory | 1.68*10-28 |
Appraisal
It is well known that Rutherford’s theory of scattering fails at high energy. On the basis of Melissinos’ experiment, we must also reject this theory at low energy. But, given the present climate of thought, Melissinos himself could not come to this conclusion. He says
(Ref. 3, p.250):
The difference between the observed and theoretical constants, while at first sight large, can be traced to the limited sensitivity of the apparatus and mainly to
(a) Uncertainty in incoming flux
(b) Uncertainty in foil thickness [and, to a lesser extent, to]
(c) Extended size of the beam and lack of parallelism
(d) Extended angular size of the detector
(e) Plural scattering in the foil (for the data at small angles)
(f) Background (for the data at large angles)
However, given the close result of the Reciprocal System, it now appears that Melissinos is too modest. Even with some uncertainty in the theoretical value of KG and the experimental values of Io and Ek, it appears that the Reciprocal System is consistent with the observed result, whereas conventional theory is not.
Can an appeal to more sophisticated mathematics rescue the current theory? It cannot. From Ref. 1 (p. 223) we have this statement:
It is a remarkable fact that a thoroughgoing wavemechanical treatment of scattering by an inversesquare force yields precisely the same result as that yielded by the strictly classical particle analysis discussed here.
Thus, despite the thousands of books, the thousands of papers, and the thousands of lectures on the nuclear theory of the atom, the physicists are going to have to discard their cherished concept.
The scattering equation of the Reciprocal System will next have to be applied to other pairs of incident and target particles and to other energy levels.
References
- R. Weidner and R. Sells, Elementary Modern Physics (Boston: Allyn & Bacon, 1968), pp. 222-223.
- H. Geiger and E. Marsden, “The Laws of Deflexion of a Particles through Large Angles, ” Philosophical Magazine, 25 (1913), 604-628.
- A. Melissinos, Experiments in Modern Physics (New York: Academic Press, 1966), pp. 231-252.
- D. Larson, The Structure of the Physical Universe (Portland, Ore.: North Pacific Publishers, 1959). (The revised edition is being published in several volumes, the first of which is Nothing But Motion, 1979).
- D. Larson, The Case Against the Nuclear Atom (Portland, Ore.:North Pacific Publishers, 1963).
- D. Larson, New Light on Space and Time (Portland, Ore.: North Pacific Publishers, 1965), pp. 115-116.
- R. Satz, “Time Region Particle Dynamics, ” Reciprocity IX.2 (Summer, 1979).
- H. Enge, M. Wehr, J. Richards, Introduction to Atomic Physics (Reading, Mass.: Addison Wesley Publishing Co., 1972).
