Chapter XIII

Into a Second Dimension

Although it may not have been apparent at the time, as soon as the compound motion principle was stated in Chapter VII and the development of the structures discussed up to that point was shown graphically on Chart A, the entire course of further development was clearly and unmistakably defined. Each additional step that we have since taken was definitely required for a logical and orderly extension of the system portrayed by the chart, and as each successive advance was made, an examination of the chart as currently revised clearly indicated what the next step must be.

Chart A was obviously incomplete at the rotational level, since we know that rotation can take place in one or two dimensions just as well as in three dimensions. Extension of the theoretical structure to include rotation in less than three dimensions then added the sub-atomic particles to the system, as indicated in Chart B. But this chart was clearly not complete either, as only three general types of motion—unidirectional translation, linear vibration, and unidirectional rotation—had been introduced up to this point, whereas a fourth—rotational vibration—was still available. Chapter XII examined the effect of addition of one-dimensional rotational vibration to the structures of Chart B and produced positive and negative ions, the charged electron, and the charged positron, as indicated on Chart C.

Now an examination of the enlarged chart shows that there is still a vacant place in the picture. Rotational vibration cannot be three-dimensional, for reasons previously explained, but two-dimensional rotational vibration is possible, and this kind of motion must therefore be added to the system. Before proceeding with this addition, however, it may be advisable to make a few comments on the general nature of the results that will be obtained from here on. Thus far, the nature and properties of the physical entities of the RS universe have not been very far out of line with currently accepted ideas as to the characteristics of the corresponding entities of the observed physical universe, The resemblance is, indeed, reasonably close in the case of the better-known phenomena such as radiation and matter. Where the conclusions of the Reciprocal System in these areas are at variance with current doctrine, scientific literature usually contains at least a few speculations somewhat along the line of the conclusions reached herein, and the new ideas are therefore not entirely unfamiliar.

As we have moved into areas where factual information is less plentiful and where existing theory is vague and confused, the agreement between existing theory and the new theoretical structure has become progressively poorer. The new electrical theory outlined in Chapters XI and XII, for instance, calls for a wholesale revision of existing ideas and concepts. Now we are about to enter some areas in which factual information is still more scarce and in which existing theory is still vaguer and more confused. In these areas the new theoretical system will diverge still farther from existing thought. The ideas developed in this present chapter will bear little resemblance to current physical theory and those in the next chapter practically no resemblance at all.

Since this almost total lack of agreement with previous thought may be hard for many scientists to accept, it should be emphasized that such divergence is inevitable. It is in these relatively new and poorly understood regions that the weaknesses of present-day theory are so clearly exposed, and it is here that the urgent call for a “drastic change in our physical picture,” a “recasting of fundamental ideas” and a “basic conceptual innovation”, originates. If we are to have a drastic change in our physical picture then there must necessarily be some drastic changes in our description of physical processes in the less clearly understood areas of physical science.

In this connection, it should be realized that the lack of adequate information from observation and experiment is not the same handicap to a purely theoretical construct like the Reciprocal System as it is to the ordinary physical theory. The usual theory is designed to explain the observations, and if the observations are limited and of uncertain accuracy, the theory is correspondingly unreliable. The Reciprocal System, on the other hand, is derived entirely from the Fundamental Postulates, and the conclusions of this system are independent of any information from observational sources. It is convenient, to be sure, to be able to check these conclusions against the results of observation at every stage of the theoretical construction as a means of keeping the chain of reasoning free from error, but in principle, this continuous checking is not necessary, and if sufficient care is taken in the logical and mathematical development the theoretical conclusions should correctly represent the physical facts not only in the areas where observational information is reasonably complete, but also in areas where it is meager and questionable, and even in areas which are as yet entirely unknown to observation. It must be conceded that where continuous checking against observation is not possible, accuracy in all details is not likely to be maintained, simply because of the limitations of human reasoning processes, but there should be no question as to the validity of the general picture derived by extension of principles whose validity has been established in the more familiar and accessible regions.

With this knowledge that we are likely to find some things considerably different from the way in which present-day physical science pictures them, let us look at an atom of matter to which has been added one or more units of two-dimensional positive rotational vibration. A one-dimensional addition of the same kind was previously identified as an electric charge and the product as a positive ion. We can expect the effect of the two-dimensional vibration to be quite similar, and it is evident that we can identify this effect with the physical phenomenon known as magnetism. The result of the addition of rotational vibration in this case has been the production of magnetized matter.

Since electric and magnetic phenomena originate in the same manner and have only one basic point of difference—the number of effective dimensions of rotational vibration—it will be appropriate to use the same terminology in application to both, and to consider the terms “electric” and “magnetic” as convenient abbreviations for the rather awkward expression “one-dimensional rotational vibratory” and its two-dimensional equivalent. Thus we will designate the two-dimensional rotational vibration as a magnetic charge.

Magnetic charges, like electric charges, can be either positive or negative, but since all two-dimensional rotation in the material universe is in time, all of the magnetic charges directly associated with matter are positive. The geometry of two-dimensional rotation is such, however, that the direction of the rotational motion relative to any external object varies with the location of that object. A rotation which is clockwise from one direction, is counterclockwise from the opposite direction. If the scalar space-time direction of the rotation is outward with respect to an object in the direction from which this rotation appears clockwise, it is inward with respect to an object in the other direction. A positive magnetic charge therefore has both positive and negative aspects, and the two centers of the directional effects, or poles, have many of the characteristics of opposite electric charges. An important difference is that a single pole does not exist by itself; there is no magnetic equivalent of the isolated electric charge. Inasmuch as the poles are merely two aspects of the same thing they always occur in pairs, and no matter how many times we may subdivide a piece of magnetized matter, each fragment has both a positive and a negative pole.

The forces exerted by magnetic charges are similar, in their general aspects, to those produced by electric charges, and also to gravitational forces. The magnitudes of all of these forces can be represented by the same equation:

F = kX₁X₂/d²

where k is a constant with a specific value in each of the three phenomena, and X₁ and X₂ represent the two (apparently) interacting quantities: masses, magnetic charges (flux) and electric charges respectively.

The identity of the force equations is to be expected since electric and magnetic charges are rotational motions of the same general character as the gravitational rotation, differing only in that they are vibrational rather than unidirectional, and in having fewer dimensions. Since the force is a scalar effect of the motion, it is immaterial whether this motion is vibrational or unidirectional, and the only significant difference between the three phenomena from the force standpoint is in the dimensions of the motion.

The existence of both positive and negative electric charges and magnetic polarities of opposite directions has generally been accepted as convincing evidence that the origin and nature of electric and magnetic phenomena must be quite different from those of gravitation, which always has the same direction in our familiar everyday world. The findings of this present work are that this conclusion is wrong on two counts. First, gravitation does not always operate in the same direction, but has different directions in different regions of the universe. One of these, the time region, in which there is motion in time within a single space unit, has already been mentioned, and other such unfamiliar regions will be discussed in Chapter XIV.

The second significant fact disclosed by the present investigation is that even in the regions where gravitation is always inward, the reversibility of magnetic and electric phenomena does not originate from any different basic characteristics; it is purely the result of dimensional differences. Obviously three-dimensional rotation does not have the kind of directional aspects that are responsible for the generation of magnetic polarities by two-dimensional rotation. Likewise, the status of matter as a compound motion with net displacement in time means that the three-dimensional motion of material atoms always has the same direction, not because rotation in the opposite direction is impossible, but because it is net rotation in the material direction that distinguishes matter from non-matter. The three-dimensional rotation of the material atom thus lacks the reversibility of the one-dimensional rotation, which makes it possible for electric charges to be either positive or negative.

In order to understand just how the electric and magnetic forces are related to the gravitational force, let us take a closer look at the direction of the gravitational motion. As brought out in the previous discussion, the gravitational motion of each individual atom is not a relation between this atom and other atoms of matter, as it seems to be, but a relation between the individual atom and the general framework of space-time. The scalar aspect of the rotational motion of the atom moves it inward in space toward all locations in space-time, in opposition to the motion of the space-time progression, which is carrying the location that the atom occupies (momentarily) outward in space away from all other locations. We cannot see space locations, but we can see objects, which occupy these locations, and we therefore note that each such object, if not restrained, moves inward in space toward all other objects.

It is important to realize, however, that what we see is only one part of the action that is taking place. We see a unit mass, which we will call object A, moving in space toward the spatial location occupied by object B but; in reality, object A is moving in space-time toward the space-time locations occupied by object B. The significance of this, in the present connection, is that if object A were moving, only toward the spatial location occupied by object B, then the mass of object B should not affect the velocity of A (the gravitational force). This, of course, contradicts experience, which indicates that the force is proportional to the mass of B. The explanation is that although the m mass units of object B occupy substantially the same spatial location, they occupy m different temporal locations, and consequently m different space-time locations. What we see as a motion toward one specific space location is actually a motion toward m different space-time locations, and its magnitude is m times as great as the portion of the total motion of object A that is directed toward a single space-time location.

A reference to the somewhat analogous relation of the gravitational force (or motion) to distance may be helpful if there is any difficulty in grasping the nature of this force to mass relationship. From the probability principles we can deduce that a scalar effect such as the gravitational force is exerted equally in all directions. It follows from geometrical considerations that at distance d the total force is distributed over a spherical surface of radius d, and the portion of the total force which is exerted against a unit area at this distance depends on the ratio of that unit area to the total area of the spherical surface. This is the familiar inverse square relation. If the unit area is so small compared to the total surface that the probability of any appreciable number of coincidences in n units selected at random is negligible, the force exerted against n units is then n times the force exerted against one unit.

If we now substitute locations in space-time for locations in space, it can easily be seen that the general situation remains the same. Again the force exerted against an individual space-time unit depends on the ratio of that unit to the total number of space-time units over which the force is distributed, and since each mass unit occupies a separate unit of space-time, even though it may be part of an aggregate of m units occupying substantially the same space location, the force exerted against these m mass units is m times the force exerted against one unit.

The general characteristics of the forces exerted by electric and magnetic charges are similar to those of the gravitational forces discussed in the preceding paragraphs; that is, an object A carrying a unit charge moves toward or away from the location occupied by another charged object B. and the motion of A, or the corresponding force, is proportional to the charge on B. The explanation of this relation is the same as that given for the analogous gravitational phenomenon. The electric and magnetic forces are, however, much stronger than the gravitational forces and this is a point that needs some further attention.

For an explanation we again turn to a consideration of the situation which exists in three-dimensional time. As already noted, the net displacement of the rotation of material atoms is in time, and when we speak of them as rotating in three dimensions, these are three dimensions of time, not dimensions of space. Time is connected with space only as a scalar quantity, and hence only one dimension of an n-dimensional motion in time can transmit its effects into space. If the motion is one-dimensional, all of the effects can be transmitted. If it is two-dimensional, the fraction transmitted into space is 1/c of the total, where c is the magnitude of unit velocity. Similarly, the transmitted fraction is only 1/c² in the case of three-dimensional rotation.

The direction in space corresponding to the scalar magnitude transmitted from the time region is indeterminate and the effects of the rotation in time are therefore distributed over three dimensions of space by probability factors irrespective of the characteristics of the time rotation. But the full effect of the one-dimensional electric rotation (or rotational vibration) is experienced in space, whereas only 1/c of the effect of the magnetic rotation and only 1/c² of the effect of the gravitational rotation is transmitted into space. Since c is the velocity of light, a very large quantity in the context of our world of everyday experience, the magnetic force is inherently much weaker than the electric force, and the gravitational force is much weaker still. Gravitational forces play such a significant role in the universe only because of the tremendous concentrations of matter which make the aggregate strength of these forces enormously greater than that of the electric and magnetic forces, in spite of the disparity in the size of the individual units.

In the light of the foregoing explanation the question now naturally arises as to why charges should act only on charges; that is, why the quantity terms q and q’ in the electric force equation (Coulomb’s Law) F=qq’/d² are limited to electrical quantities. If the charge is actually a motion of the same general nature as gravitation, it should be a motion toward all space-time locations, just as gravitation is, and it should therefore exert a force not only on charged objects but on all objects. The answer is that it does exert a force on all objects, but since the force exerted on an uncharged mass is only 1/c² as great as the force on an object with a charge of comparable magnitude, the effect on uncharged matter is too small to be significant. Similarly, magnetic charges (magnetized matter) not only exert forces on other magnetic charges but also exert analogous, though much smaller, forces on all matter. The familiar classification of material substances under the designations paramagnetic and diamagnetic is made on the basis of this relatively weak magnetic effect.

Inasmuch as radiation has both electric and magnetic aspects, as the term electromagnetic radiation implies, it has been taken for granted in current scientific thought that the effects produced by electric and magnetic charges are propagated by means of this radiation. Then, since the gravitational force bears such a close relationship to the electric and magnetic forces, it has been further assumed that the gravitational effects must be propagated in a similar manner and a great deal of effort has been devoted to a wholly unsuccessful search for evidence of “gravitational waves.” The findings of this work now show that the physicists are correct in their conclusion that electric, magnetic and gravitational forces are phenomena of the same general nature and therefore behave in a similar manner, but these findings also indicate that instead of all of these forces being propagated in the same manner, as current thought assumes, none of them is propagated in any manner. Like the gravitational motion, the motion which gives rise to the electric or magnetic forces is a motion of the individual atom or particle with respect to the general structure of space-time, not an action of this unit on some other charged or uncharged object as it appears to be. And like gravitation, the electric and magnetic forces are therefore effective instantaneously; they are not propagated by radiation or by any other mechanism. Electromagnetic radiation is a phenomenon of a related but quite different character.

Another of the many instances where a relationship between phenomena has been mistaken for evidence of identity of the phenomena can be found in electromagnetism. It has been observed that electric charges in motion produce magnetic effects and that electric currents produce similar effects. Mainly on the basis of these observations, physicists have concluded (1) that electric currents are electric charges in motion, and (2) that moving electric charges are the essence of magnetism. In the words of a physics textbook, “magnetic forces are forces associated with the motion of electric charges.”¹¹⁴

Absence of the electric forces that would be expected to exist, on the basis of this hypothesis, in magnetized matter and in conductors carrying currents is a serious weakness in the hypothesis that is glossed over in current practice. The explanation currently offered is that the negative charges of the electrons are “exactly equal and opposite to the fixed positive charge” in the conductor, thus making the conductor electrically neutral. There are many contradictions and inconsistencies in such a hypothesis which current theory simply ignores. For example, the hypothesis requires the electric charges to be firmly fixed, so that they can account for the strong cohesive forces between the atoms of the conductor, and yet easily movable, so that they can account for the ready response of the current to any impressed potential. Furthermore, an “exact” equality between the negative charges of the current and the “fixed positive charge” of the atoms of the conductor is assumed in order to account for the fact that a wire carrying a current is electrostatically neutral, but then in order to account for differences in potential it is assumed that there is an “excess of electrons” in the regions of higher potential which “may be supposed to produce a sort of electron pressure within the metal.”¹¹⁵ And above all, there is the very awkward fact, previously mentioned, that a flow of static charges, which is known to be a movement of charges or charged particles, has some characteristics which are quite different from those of a flow of electric current, which is assumed to be a movement of charges or charged particles, and it can readily be distinguished from the latter.

The confusion in the electric current situation was cleared up in Chapter XI by showing that the Reciprocal System requires the current to be a flow of uncharged rather than charged electrons. But this cuts the ground out from under the accepted explanation of the origin and nature of magnetism, and at this time, therefore, we will want to determine what information the new theoretical development can give us about magnetic fundamentals. As we have seen, the electric charge is a one-dimensional modification of the rotational motion of an atom or sub-atomic particle and the magnetic charge is a similar two-dimensional modification. The characteristic effects of the magnetic charge originate because the one-dimensional forces are distributed over two dimensions by the second rotation. But for this purpose it is not necessary that the motion in the second dimension be rotational. We can see why this is true if we examine the behavior of the axes of rotation. The axis of the electric rotation of an atom is a line: a one-dimensional figure. A stationary electric charge thus has no two-dimensional rotational effects. For a magnetic charge the locus of all positions of either axis is a disk: a two-dimensional figure, and the magnetic charge has two-dimensional properties. But if we move the electric charge translationally, the locus of all positions of the axis is again a two-dimensional figure, and hence a moving electric charge has a two-dimensional distribution of forces comparable to that of a magnetic charge.

The basic rotational motions of atoms and sub-atomic particles generate only gravitational forces, as they are distributed over three time dimensions even where the motion itself takes place in less than three dimensions. In their normal states, therefore, these units have neither electric nor magnetic properties. When an atom or particle is given a rotational vibration, the scalar direction of this motion necessarily opposes that of one of the rotational motions but there is no requirement that the spatial and temporal directions maintain any specific relation, and the full scalar effect of one dimension of rotational vibration is therefore transmitted into space. An electrically charged unit, an ion or a charged particle, thus produces one-dimensional, or electric, effects.

Similarly, a magnetically charged unit, which has rotational vibration in two dimensions, produces two-dimensional, or magnetic, effects. If an uncharged electron or positron is given a translational motion, this again is motion in two dimensions and it produces electromagnetism, a magnetic effect. An uncharged atom in motion produces a similar effect, to which the term gyromagnetism is applied, as it has thus far been observed only in rapidly rotating objects. If a charged electron is given a translational motion, the compound motion is still only two-dimensional, and the magnetic situation is the same as if the electron were uncharged, but the electric force of the charge is also effective, hence the moving charged electron produces both electric and magnetic effects.

We have now gone about as far in, the discussion of ordinary magnetic phenomena as can be justified in a brief general survey of this kind, but there is another magnetic effect of a somewhat different nature, not hitherto recognized as having any connection with magnetism, which we will want to examine. In order to lay the groundwork for an explanation of this phenomenon, let us give some consideration to the elusive particle known as the neutrino. According to the findings of the Reciprocal System, the neutrino is one of the five possible sub-atomic particles of the material system. This particular particle has one effective unit of positive two-dimensional rotation and one of negative one-dimensional rotation. The two oppositely directed motions neutralize each other from the scalar space-time standpoint, hence the net space-time displacement of this rotational combination is zero. With both one-dimensional and two-dimensional rotations, this particle is capable of taking either an electric or a magnetic charge but, on the basis of probability considerations, the magnetic charge takes precedence, and under appropriate conditions the neutrino acquires a one-unit positive magnetic charge. This is a unit space displacement, and since the neutrino is otherwise featureless, the charged neutrino is essentially nothing but a mobile unit of space, similar, in this respect, to the uncharged electron. Like the latter, it can move freely in matter, but is barred from motion through space, simply because the relation of space to space is not motion.

Neutrinos are produced in substantial quantities in some common physical processes and since they move freely through either space or matter when in the uncharged condition, each body in the universe is subjected to a continuous flux of neutrinos in much the same way that it is subjected to a continuous bombardment by photons of radiation. Occasionally one of these neutrinos acquires a charge in passing through matter, and when this occurs the neutrino is trapped and cannot escape. The concentration of charged neutrinos in matter therefore builds up, as the material grows older.

The difference between the situation of the charged neutrino and that of the uncharged electron should be specifically noted. While these two particles are analogous to the extent that each is a unit of space and hence can move only through matter, the uncharged electron can escape from this limitation by acquiring a charge, and a continued build-up of the concentration of these electrons also builds up forces which will ultimately become strong enough to produce the required charge. The charged neutrino, on the other hand, can escape only by losing its charge, and since here also a continued build-up of the concentration of these particles builds up forces tending to produce charges, the possibility of losing a charge becomes more remote as the concentration increases.

In order to appreciate the significance of this build-up it is necessary to recognize that the reciprocal relation between space and time makes any motion of a particle with reference to the atom in which it is located equivalent to an oppositely directed motion of the atom with reference to the particle. Inasmuch as these motions are equivalent, they reach equilibrium. Thus the thermal motion of the uncharged electrons is equivalent to and in equilibrium with the oppositely directed thermal motion of the atoms in which they are located. In the situation we are now considering the rotational vibration of the neutrinos is similarly equivalent to and in equilibrium with an oppositely directed rotational vibration of the atoms in which they are located. Since the charge of the neutrino is a magnetic space displacement its presence causes the atom to acquire a magnetic charge with a time displacement. This is opposite in space-time direction to the usual magnetic charge, a seemingly minor point of difference, but one which, in this case, has some far-reaching consequences.

The ordinary magnetic charge is foreign to the material environment, a two-dimensional space displacement in a structure whose very essence is a net time displacement, and it therefore plays a relatively minor role in the phenomena of the material universe. The oppositely directed charge of the same nature, on the other hand, is a motion identical with the basic two-dimensional rotation of the atom, except that it is vibratory rather than unidirectional. Consequently it adds to and, in a sense, merges with, the atomic rotation, and has the same general effect as an equivalent addition of rotational displacement.

Instead of exhibiting a behavior of a different kind, such as that which distinguishes an ion or a magnetized particle from a particle of ordinary matter, the two-dimensional charge due to the presence of the charged neutrino simply adds to the magnitudes of the normal properties of the atoms. For this reason we will not use the term “magnetic charge” in referring to this motion, but will call it a “gravitational charge.” The most conspicuous effect of the gravitational charge is an increase in the mass of the atom. Because of its vibrational character each unit of this charge is only half as effective as a unit of unidirectional rotation and, for convenience, the mass due to this half unit of effective displacement has been adopted as the unit of atomic weight. The atomic weight of the normal atom is thus twice the atomic number Z (the number of effective notational displacement units) and each unit of gravitational charge adds one unit of atomic weight. Since the number of units of charge which the atoms may acquire is variable, each normal atom of atomic weight 2Z is accompanied by a series of isotopes with isotopic weight 2Z + G.

In our local environment the various isotopes of each chemical element usually occur in fixed proportions and the average isotopic weight of the element is recognized as the atomic weight of that element. It is evident from the foregoing discussion, however, that the existing isotopic proportions are not inherent in the structure of matter itself but are results of the magnetic ionization level prevailing in the local environment. In a location where the magnetic ionization level is different, the isotopic proportions will also be different. We actually have some evidence of this locally, as some variability in atomic weights has been observed. For instance, the atomic weight of lead from various sources differs slightly.

We can deduce from the theoretical principles involved that in very young matter, where the magnetic ionization level is zero or near zero, there are no isotopes, and the atomic weight of each chemical element is its rotational value 2Z. Here all of the rotational combinations (elements and sub-atomic particles) that are possible, all the way from the electron to element, 117 are stable. In this young matter heavier elements are continually being built up from lighter ones by a process of neutron capture, and there is no destruction or degradation of an element once produced, unless the limiting atomic weight of 236 (the atomic weight of the unstable element 118) is reached.

If this matter is now transferred to a region of higher ionization level, such as the surface of the earth in its present condition, some of the atoms will acquire gravitational charges. From theoretical considerations it has been determined that at any given magnetic ionization level, the normal increase in mass due to acquisition of gravitational charges in the process of attaining equilibrium with the charges of the neutrinos varies as the square of the atomic weight of the uncharged atom. A quantitative evaluation, previously published, also reveals that at a one-unit ionization level, which is approximately the level of the local environment, the normal atomic weight increment varies from practically zero for the lowest elements to 3 for element 20, 10 for element 40, 23 for element 60, 41 for element 80, 54 for element 92, and so on. When the 54 increment is added to the 184 atomic weight of the normal atom of element 92, the total becomes 238, which is above the 236 limit. In this local environment, therefore, element 92, uranium, and all above it are theoretically unstable and will disintegrate by ejection of mass. Some of the elements immediately below number 92 can also exceed the stability limit because of a probability distribution factor similar to that which permits evaporation at relatively low average temperatures.

This theoretical disintegration process which takes place in the RS universe can obviously be correlated with the observed phenomenon which we call radioactivity. On first consideration, however, there appears to be a discrepancy between the theoretical characteristics of the process and those, which are actually observed. The derivation of the theoretical disintegration clearly requires it to be an explosion: a single event initiated as soon as an aggregate reaches the stability limit and continuing until the process is complete. The observed radioactivity, on the other hand, seems to be a series of independent events occurring at random within the aggregate, and often extending over a very long period of time. The “half-life” of some of the isotopes of uranium, for instance, runs into millions or even billions of years.

In the context of present-day physics, these two descriptions are wholly irreconcilable, but in the Reciprocal System the radioactive explosion is simply the inverse of an ordinary explosion; that is, it is the same process with space and time interchanged. In an ordinary explosion, the action begins at one or more points in the aggregate and is propagated outward in space from these points at a high velocity. Each atom of the aggregate remains in its original state until the progress of the action reaches the location in space, which this atom occupies, whereupon it suddenly disintegrates. The explosion as a whole therefore takes the form of a series of individual explosions at different locations in space initiated successively by an agency propagated through space at a finite velocity. In a radioactive explosion, the action begins at one or more points in the aggregate and is propagated outward in time from these points at a high inverse velocity (that is, slowly). Each atom of the aggregate remains in its original state until the progress of the action reaches the location in time, which this atom occupies, whereupon it suddenly disintegrates. The explosion as a whole therefore takes the form of a series of individual explosions at different locations in time initiated successively by an agency propagated through time at a finite velocity. Aside from substituting time for space, this description of the radioactive explosion is identical with the preceding description of the ordinary explosion.

To those who are steeped in the traditional ideas of physics, this explanation of radioactivity will no doubt appear as a wild flight of fancy. The conclusive answer to any such suggestion is the standard argument based on the analogy of the aerial map. Inasmuch as we have demonstrated the validity of the Reciprocal System as a whole by comparisons in many other fields where accurate comparisons are possible, and since there is no inconsistency between the known facts of radioactivity and the conclusions of the new system, there is no logical basis from which these conclusions can legitimately be challenged. The accuracy of an aerial map cannot be impeached on the ground that someone thinks there is a mountain where the map shows a lake, nor can the propagation of an explosion through time be denied on the similar ground that physicists have never heard of such a thing before.

Furthermore, it should be pointed out that even though the absence of any contradictory evidence is sufficient to establish the validity of the new theory of radioactivity, in view of the general proof of the Reciprocal System as a whole, in this instance we do not have to rely solely on the lack of any contradiction. The fact is that every feature of the theoretical radioactive explosion, with only one exception, can be verified by observation. The theory says that the explosion as a whole consists of a series of successive atomic explosions, and this is exactly what we observe. The theory says that the action will, in general, proceed very slowly, and that the time interval between successive explosions will vary over an extremely wide range, depending on the characteristics of the substance undergoing the explosion. We observe that this is true. The theory says that the action, once initiated, will continue to completion, just as an ordinary explosion does, and this is what we find happening. The only thing that we cannot verify in any direct manner is the theoretical conclusion that the action is propagated through time rather than through space. Here, of course, we must rely on the proof of the validity of the system as a whole.

This novel explanation of the seemingly haphazard series of events in radioactivity, number twelve in the series of Outstanding Achievements described in this volume, is only one of the many simple, but wholly time expected, answers that the Reciprocal System has provided for problems that, according to current scientific thought, have no answers. “There are no physical laws to tell us—and there cannot be,”¹¹⁶ says Bronowski, with reference to this radioactivity problem. From the point of view of wave mechanics, reports Capek, “radioactive explosions are regarded as contingent events whose irreducible chance character manifests the basic indeterminacy of microphysical occurrences.”¹¹⁷ Whittaker is no less positive: “Thus the accurate prediction of the moment of the explosion is impossible not only in present practice but in eternal principle, and the statistical or probability law must be accepted as a primary law of nature, which can never be superseded by a more fundamental law of a deterministic character.”¹¹⁸

But the Reciprocal System now provides the physical laws which Bronowski says cannot exist, it eliminates the element of chance which Capek says is irreducible, and it demolishes Whittaker’s “eternal principle,” again demonstrating the error in the conclusion that the ultimate processes of nature are not “understandable and subject to law.” Once more we find that the real difficulty is that the experimenters have outstripped the theorists and have penetrated into regions of the universe which the theorists are totally unprepared to deal with. When the horizons of theory are properly widened, the universe is once more seen in its true character as orderly and rational.

In their appraisal of radioactivity, present-day theorists have been unable to see any spatial relation between one decay event and the next, hence they have concluded that there is no relation of any kind, “and there cannot be,” as Bronowski puts it. Current theory simply cannot visualize the possibility that a succession of events which is random from a spatial standpoint may be quite orderly from some other standpoint. But this is just the situation that exists in the RS universe, and therefore, by reason of the demonstrated identity between this theoretical universe and the observed physical universe, exists in the latter as well. Atoms, which are contiguous in space, may be, and usually are, widely separated in coordinate time. Conversely, atoms, which are far apart in space, may be contiguous in coordinate time. What the new theory tells us is that the radioactive explosion is propagated in coordinate time in the same manner that a gas explosion is propagated in coordinate space. The order in which the successive atoms are affected is just as systematic in radioactivity as in the gas explosion, but it is based on temporal proximity, not on spatial proximity. The reason why the radioactive explosion takes the temporal rather than the spatial form will be explained in the next chapter.

It should be understood that this concept of an action being transmitted from one atom to another that is contiguous in time rather than in space has nothing to do with clock time, the only kind of time recognized prior to the development of the Reciprocal System. A gas explosion, in which the action is transmitted from one atom to another that is contiguous in space, is not affected in any way by the change in the position of these atoms due to the recession of the galaxy, the movement which is carrying it away from all other galaxies: a motion in clock space, as it has been termed in this work. This explosion is propagated in coordinate space, the extension space of our everyday experience. Similarly, the radioactive explosion is propagated in coordinate time, the hitherto unrecognized kind of time analogous to coordinate space that was discussed in Chapter VIII, and it is not affected by the position of the radioactive material in clock time, the familiar kind of time that is analogous to the clock space: the space of the galactic recession.

Although it is not generally recognized, there are two very different types of radioactivity. The one that has been discussed thus far originates when the total mass of an atom exceeds the atomic weight limit 236, and it is characterized by an ejection of mass, usually in the form of alpha particles (helium atoms). We will therefore designate this as alpha radioactivity. Another somewhat similar process occurs when the ratio of vibrational to rotational mass deviates too far from the normal value established by the prevailing magnetic ionization level. For example, the normal vibrational mass for element 40, zirconium, at unit ionization level, is 10, as previously stated. The normal isotopic weight corresponding to this ionization level is then 90. Since atoms are inherently quite stable structures, this normal isotope is not usually the only stable one, nor is it necessarily the most abundant (although in the case of zirconium it happens to be); it is the approximate center of a zone of isotopic stability, the width of which varies from element to element.

Isotopes within this zone are stable, as a rule. Zirconium has stable isotopes at 90, 91, and 92. Outside the stability zone the isotopes are generally unstable and are subject to processes, which move them toward the normal isotopic weight. Below the zone of stability the process is ejection of positrons, or some equivalent. Above the zone of stability the movement toward the stable zone requires an action of the opposite character, and the process is ejection of electrons or the equivalent. The electrons and positrons emitted in these radioactive processes are known as beta particles, and in the present series of publications these processes will be designated as beta radioactivity. The loss of mass in alpha emission often puts the atom outside the stability zone, requiring beta radioactivity for correction of the mass ratio. The heavier radioactive elements therefore decay in a series of steps in which alpha and beta radioactivity follow each other in a rather irregular succession.

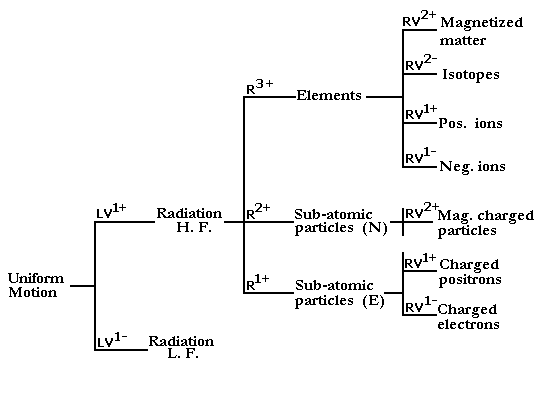
Chart D

The addition of isotopes and the other new entities introduced earlier in the chapter, magnetically charged sub-atomic particles and magnetized matter, to Chart C completes the upper or material branch of the compound motion system, as the chart now covers all four of the basic types of motion and all of the dimensional variations that are possible for each of these types. Chart D shows how the system looks after these additions are made.