CHAPTER 18
Simple Compounds
In the preceding chapters we have determined the specific combinations of simple rotations that are stable in the material sector of the universe, and we have identified each of these combinations, within the experimental range, with an observed sub-atomic particle or atom of an element. We have then shown that an exact duplicate of this system of material rotational combinations, with space and time interchanged, exists in the cosmic sector, and we have identified all of the observed particles that do not belong to the material system as atoms or particles of the cosmic system. To the extent that observational or experimental data are available, therefore, we have established agreement between the theoretical and observed structures. So far as these data extend, there are no loose ends; all of the observed entities have been identified theoretically, and while not all of the theoretical entities have been observed, there are adequate theoretical explanations for this.
The number of observed particles is increased substantially by a commonly accepted convention which regards particles of the same kind, but with different electric charges, as different particles. No consideration has been given to the effects of electric charges in this present discussion, as the existence of such charges has no bearing on the basic structure of the units. These charges may play a significant part in determining whether or not certain kinds of reactions take place under certain circumstances, and may have a major influence on the details of those reactions, just as the presence or absence of concentrations of kinetic energy may have a material effect on the course of events. But the electric charge is not part of the basic structure of the atom or sub-atomic particle. As will be brought out when we take up consideration of electrical phenomena, it is a temporary appendage that can be attached or removed with relative ease. The electrically charged atom or particle is therefore a modified form of the original rotational combination rather than a distinctly different type of structure.
Our examination of the basic structures is not yet complete, however, as there are some associations of specific numbers of specific elements that are resistant to dissociation, and therefore act in the manner of single units in processes of low or moderate energy. These associations, or molecules, play a very important part in physical activity, and in order to complete our survey of the units of which material aggregates are composed we will now develop the theory of the structure of molecules, and will determine what kinds of molecules are theoretically possible.
The concept of the molecule originated from a study of the behavior of gases, and as originally formulated it was essentially empirical. The molecule, on this basis, is the independent unit in a gas aggregate. But this definition cannot be applied to a solid, as the independent unit in a solid is generally the individual atom, or a small group of atoms, and in this case the molecule has no physical identity. In order to make the molecule concept more generally applicable, therefore, it has been redefined on a theoretical rather than an empirical basis, and as now conceived, a molecule is the smallest unit of a substance which can (theoretically) exist independently and retain all of the properties of the substance.
The atoms of a molecule are held together by inter-atomic forces, the nature and magnitude of which will be examined in detail later. The strength of these forces determines whether or not the molecule will break up under whatever disruptive forces it may be subjected to, and the manner in which certain atoms are joined in a molecule may have an effect on the magnitude of the inter-atomic forces, but the determination of what atoms can combine with what other atoms, and in what proportions, is governed by an entirely different set of factors.
In current theory, the factors responsible for the inter-atomic force, or “bond,” are presumed to have a double function, not only determining the strength of the cohesive force, but also determining what combination can take place. The results of the present investigation indicate, however, that the force which determines the equilibrium distance between any two atoms is identical in origin and in general character regardless of the kind of atoms involved, and regardless of whether or not those atoms can, or do, take part in the formation of a molecule.
Experience has indicated that it is advisable to lay more emphasis on the independence of these two aspects of the interrelations between atoms, and for this purpose the plan of presentation employed in the first edition will be modified in some respects. As already mentioned, the information that will be developed with respect to the molecular structure will be presented before any discussion of inter-atomic forces is undertaken. Furthermore, present indications are that whatever advantages there may be in using the familiar term “bond” in describing the various molecular structures are outweighed by the fact that the term “bond” almost inevitably implies the notion of a force of some kind. Inasmuch as the different molecular “bonds” merely reflect different relative orientations of the rotations of the interacting atoms, and have no force implications, we will abandon the use of the term “bond” in this sense, and will substitute “orientation” for present purposes. The term “bond” will be used in a different sense in a later chapter where it will actually relate to a force.
The existence of molecules, either combinations of specific numbers of like atoms, or chemical compounds, which are combinations of unlike atoms, is due to the limitations on the establishment of inter-atomic equilibrium that are imposed by the presence of motion in time in the electric dimension of the atoms of certain elements. Those elements whose atoms rotate entirely in space (positive displacement in all rotational dimensions), or which are able to attain the all-positive status by reorientation on the 8-x basis, are not subject to any such limitations. An atom of an element of this kind can establish an equilibrium with any other such atoms in any proportions, except to the extent that the physical properties of the elements involved (such as the melting points) or conditions in the environment (such as the temperature) interfere. Material aggregates of this kind are called mixtures. In some cases, where the mixture is homogeneous and the composition is uniform, the term alloy is applied.
There is a class of intermetallic compounds, in which these positive constituents are combined in definite proportions. CuZn and Cu5Zn8 are compounds of this class. But the combinations of copper and zinc are not limited to specific ratios of this kind in the way in which the composition of true chemical compounds is restricted. The commercially important alloys of these two metals extend through the entire range from a brass with 90 percent copper and 10 percent zinc to a solder with 50 percent of each constituent, and the possible alloys extend over a still wider range. The intermetallic compounds are merely those alloys whose proportions are especially favorable from a geometric standpoint. A typical comment in a chemistry textbook is that “The theory of the bonding forces involved in these intermetallic compounds is very complex and is not, as yet, very well understood.” The reason is that there are no “bonding forces” in these substances in the same sense in which that term is ordinarily used in application to the true chemical compounds.
As has been stated, negative rotation in the electric dimension of an atom is admissible because the requirement that the net total rotational displacement must be positive (in the material sector) can be met as long as the magnetic rotation is positive. In the time region inside unit distance, however, the electric and magnetic rotations act independently. Here the presence of a randomly oriented electric rotation in time makes it impossible to maintain a fixed inter-atomic equilibrium. Any relation of space to time is motion, and motion destroys the equilibrium. But an equilibrium can be established in certain cases if both of the interacting atoms are specifically oriented along the line of interactions in such a manner that the negative displacement in the electric dimension of one atom is counterbalanced by an equal positive displacement in one of the dimensions of the second atom, so that the magnitude of the resulting relative motion is zero with respect to the natural datum. Or a multi-atom group equilibrium may be established where the total negative displacements of the atoms with electric rotation in time are exactly equal to the total effective positive displacements of the atoms with which the interaction is taking place.
In these cases there is an equilibrium because the net total of the positive and negative displacement involved is zero. Alternatively, the equilibrium may be based on a total of 8 or 16 units, since, as we have found, there are 8 displacement units between one zero point and the next. A negative displacement x may be counterbalanced by a positive displacement 8-x, the net total being 8, which is the next zero point, the equivalent of the original zero.
As an analogy, we may consider a circle, the circumference of which is marked off into 8 equal divisions. Any point on this circle can be described in either of two ways: as x units clockwise from zero, or as 8-x units counter-clockwise from 8. A distance of 8 units clockwise from zero is equivalent to zero. Thus a balance between x and 8-x, with the midpoint at 8, is equivalent to a balance between x and -x, with the midpoint at zero. The situation in the inter-atomic space-time equilibrium is similar. As long as the relative displacement of the two interacting motions, the total of the individual values, amounts to the equivalent of any one of the zero points, the system is in equilibrium.
Because of the specific requirements for the establishment of equilibrium, the components of combinations of this kind, molecules of chemical compounds, exist in definite proportions, each n atoms of one component being associated with a specific number of atoms of the other component or components. In addition to the constant proportions of their components, compounds also differ from mixtures or alloys in that their properties are not necessarily similar to those of the components, as is generally true in the all-positive combinations, but may be of an altogether different nature, as the resultant of a space-time equilibrium of the required character may differ widely from any of the effective rotational values of the individual elements.
The rotational displacement in the dimension of interaction determines the combining power, or valence, of an element. Since the negative displacement is the foreign component of the material molecule that has to be counterbalanced by an appropriate positive displacement to make the compound possible, the negative valence of an element is the number of units of effective negative displacement that an atom of that element possesses. It follows that, with some possible exceptions that will be considered later, there is only one value of the negative valence for any element. The positive valence of an atom in any particular orientation is the number of units of negative displacement which it is able to neutralize when oriented in that manner. Each element therefore has a number of possible positive valences, depending on its rotational displacements and the various ways in which they can be oriented. The occurrence of these alternate orientations is largely dependent upon the position of the element within the rotational group, and in preparation for the ensuing discussion of this subject it will be advisable, for convenient reference, to set up a classification according to position.
Within each of the rotational groups the minimum electric displacement for the elements in the first half of the group is positive, whereas for those in the latter half of the group it is negative. We will therefore apply the terms electropositive and electronegative to the respective halves. It should be understood, however, that this distinction is based on the principle that the most probable orientation in the electric dimension considered independently is that which results in the minimum displacement. Because of the molecular situation as a whole, an electronegative element often acts in an electropositive capacity—indeed, nearly all of them take the positive role in chemical compounds under some conditions, and many do so under all conditions—but this does not affect the classification that has been defined.
There are also important differences between the behavior of the first four members of each series of positive or negative elements and that of the elements with higher rotational displacements. We will therefore divide each of these series into a lower division and an upper division, so that those elements with similar general characteristics can be treated together. This classification will be based on the magnitude of the displacement, the lower division in each case including the elements with displacements from 1 to 4 inclusive, and the upper division comprising those with displacements of 4 or more. The elements with displacement 4 belong to both divisions, as they are capable of acting either as the highest members of the lower divisions or as the lowest members of the upper divisions. It should be recognized that in the electronegative series the members of the lower divisions have the higher net total positive displacement (higher atomic number).
For convenience, these divisions within each rotational group will be numbered in the order of increasing atomic number as follows:
These are the divisions which were indicated in the revised periodic table in Chapter 10. As will be seen from the points developed in the subsequent discussion, the division to which an element belongs has an important bearing on its chemical behavior. Including this divisional assignment in the table therefore adds substantially to the amount of information that is represented.
Where the normal displacement x exceeds 4, the equivalent displacement 8-x is numerically less than x, and therefore more probable, other things being equal. One effect of this probability relation is to give the 8-x positive valence preference over the negative valence in Division III, and thereby to limit the negative components of chemical compounds to the elements of Division I
| Division I | Lower electropositive |
| Division II | Upper electropositive |
| Division III | Upper electronegative |
| Division IV | Lower electronegative |
V, except in one case where a Division III element acquires the Division IV status for reasons that will be discussed later.
When the positive component of a compound is an element from Division I, the normal positive displacement of this element is in equilibrium with the negative displacement of the Division IV element. In this case both components are oriented in accordance with their normal displacements. The same is true if either or both of the components is double or multiple. We will therefore call this the normal orientation. The corresponding normal valences are the positive valence (x) and the negative valence (-x).
It is theoretically possible for any Division I element to form a compound with any Division IV element on the basis of the appropriate normal valences, and all such compounds should be stable under favorable conditions, but whether or not any specific compound of this type will be stable under the normal terrestrial conditions is determined by probability considerations. An exact evaluation of these probabilities has not yet been attempted, but it is apparent that one of the most important factors in the situation is the general principle that a low displacement is more probable than a high displacement. If we check the theoretically possible normal valence compounds against the compounds listed in a chemical handbook, we will find nearly all of the low positive-low negative combinations in this list of common compounds. The low positive-high negative, and the high positive-low negative combinations are much less fully represented, while we will find the high positive-high negative combinations rather scarce.
The geometrical symmetry of the resulting crystal structure is the other major determinant. A binary compound of two valence four elements (RX), for example, is more probable than a compound of a valence four and a valence three element (R3X4). The effect of both of these probability factors is accentuated in Division II, where the displacements corresponding to the normal valence have the relatively high values of 5 or more. Consequently, this valence is utilized only to a limited extent in this division, and is generally replaced by one of the alternative valences.
Inasmuch as the basic requirement for the formation of a chemical compound is the neutralization of the negative electric displacement, the alternative positive valences are simply the results of the various ways in which the atomic rotation can be oriented to attain an effective positive displacement that will serve the purpose. Since each type of valence corresponds to a particular orientation, the subsequent discussion will be carried on in terms of valence, the existence of a corresponding orientation in each case being understood.
The predominant Division III valence is based on balancing the 8-x displacement (positive because of the zero point reversal) against the displacement of the negative component. The resulting relative displacement is 8, which, as explained earlier, is the equivalent of zero. We will call this the neutral valence. This valence also plays a prominent part in the purely Division IV compounds.
The higher Division III members of Groups 4A and 4B are unable to utilize the 8-x neutral valence because for these elements the values of 8-x are less than zero, and therefore meaningless. instead, these elements form compounds on the basis of the next higher equivalent of zero displacement. Between the 8-unit level and this next zero equivalent there are two effective initial units of motion, as well as an 8-unit increment. The total effective displacement at this point is therefore l8, and the secondary neutral valence is 18-x. A typical series of compounds utilizing this valence, the oxides of the Division III elements of Group 4A, consists of HfO2, Ta2O5, WO3, Re2O7, and OSO4.
Symmetry considerations favor balancing two electric displacements to arrive at the necessary space-time equilibrium, where conditions permit, but where the all-electric orientation encounters difficulties, it is possible for one of the magnetic rotations to take the positive role in the inter-atomic equilibrium. The magnetic valences, which apply in these magnetic-electric orientations, are the most common basis of combination in Division II, where the positive valences are high, and the neutral valences are excluded because the 8-x displacement is negative. They also make their appearance in the other three divisions where probability considerations permit.
Each element has two magnetic rotations and therefore has two possible first order magnetic valences. In alternate groups the two rotations are equal, where no environmental influences are operative, and on this basis the number of magnetic valences should be reduced to one in half of the groups. As we saw in our original consideration of the atomic rotation in Chapter 10, however, any element can rotate with an addition of positive electric rotational displacement to the appropriate magnetic rotation, or with an addition of negative electric rotational displacement to the next higher magnetic rotation. Because of this flexibility, the limitation of the elements of alternate groups to a single magnetic valence actually applies only to the elements of Division I. Here this restriction has no real significance, as the elements of this division make little use of the magnetic valence in any event, because of the high probability of the low positive valences.
To distinguish between the two magnetic valences, we will call the larger one the primary magnetic valence, and the smaller one the secondary magnetic valence. Neither of these valences has any inherent probability advantage over the other, but the geometrical considerations previously mentioned do have a significant effect. For instance, where the magnetic valence can be either two or three, a combination with a valence three negative element takes the form R3X2 if the magnetic valence is two, and the form RX if the alternate valence prevails. The latter results in the more symmetrical, and hence more probable, structure. Conversely, if the negative element has valence four, the R2X structure developed on the basis of a magnetic valence of two is more symmetrical than the R4X4 structure that results if the magnetic valence is three, and it therefore takes precedence.
Many of the theoretically possible magnetic valence compounds that are on the borderline of stability, and do not make their appearance as independent units, are stable when joined with some other valence combination. For example, there are three theoretically possible first order valence oxides of carbon: CO2 (positive electric valence), CO (primary magnetic valence), and C2O (secondary magnetic valence). The first two are common compounds. C2O is not. But there is another well-known compound, C3O2, which is obviously the combination CO C2O. As we will see later, this ability of the less stable combinations to participate in complex structures plays an important role in compound formation.
The first order valences of the elements, the valences that have been discussed thus far, are summarized in Table 7. The great majority of the true chemical compounds of all classes are formed on the basis of these valences.
There is also an alternate type of inter-atomic orientation that gives rise to what we may call second order valences. As has been emphasized in the previous discussion, an equilibrium between positive and negative
rotational displacements can take place only where the net resultant is zero, or the equivalent of zero, because any value of the space-time ratio other than unity (zero displacement) constitutes motion, and makes fixed equilibrium positions impossible. In the most probable condition, the initial level from which each rotation extends is the same zero point, or, where the nature of the orientation requires different zero points, the closest combination that is possible under the circumstances. This arrangement, the basis of the first order valences, is clearly the most probable, but it is not the only possibility.
Inasmuch as the separation between natural zero points (unit speed levels) is two linear units (or eight three-dimensional units) it is possible to establish an equilibrium in which the initial level of the positive rotation (the positive zero) is separated from the initial level of the negative rotation (the negative zero) by two linear units. The effect of this separation on the valence is illustrated in Figure 2.
Figure 2
| (a) | (b) | (c) |
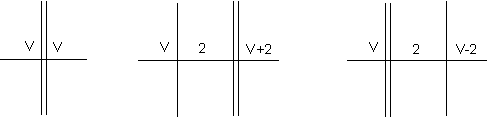
The basis of the first order valences is shown in (a). Here the normal positive valence V balances an equal negative valence V at an equilibrium point represented by the double line. In (b) the initial level of the positive rotation has been offset to the next zero point, two units distant from the point of equilibrium. These two units, being on the positive side of the equilibrium point, add to the effective positive displacement, and the positive valence therefore increases to V+2; that is, V+2 negative valence units are counterbalanced. In (c) it is the initial level of the negative rotation that has been offset from the point of equilibrium. Here the two intervening units add to the effective negative displacement, and the positive valence decreases to V-2, as the V units of positive displacement are now able to balance only V-2 negative valence units.
By reason of the availability of the zero point modifications illustrated in Figure 2(b), each of the positive first order valences corresponds to a second order valence, an enhanced valence, as we will call it, that is two units greater in the case of the direct valences (x+2), and two units less for the inverse valences: 8 - (x+2) = 6-x. Compounds based on enhanced normal valences are relatively uncommon, as the normal valence itself has a high degree of probability, and the enhanced valence is not only inherently less probable, but also has a higher effective displacement in any specific application, which decreases the relative probability still further. The probability factors are more favorable for the enhanced neutral valence, as in this case the effective displacement is less than that of the corresponding first order valences. The compounds of this type are therefore more numerous, and they include such well-known substances as SO2 and PCI3. An interesting application of this valence is found in ozone, which is an oxide of oxygen, analogous to SO2.
It should theoretically be possible for valences to be diminished by orientation in the manner shown in Figure 2(c), but it is doubtful if any stable compounds are actually formed on the basis of diminished electric valences. The reason for their absence is not yet understood. The magnetic valences are both enhanced and diminished. Either the primary or the secondary valence may be modified, but since enhancement is in the direction of lower probability (higher numerical value) the number of common compounds based on the enhanced magnetic valences is relatively small. Diminishing the valence improves the probability, and the diminished valence compounds are therefore more plentiful in the rotational groups in which they are possible (those with primary magnetic valences above two), although the list is still very modest compared to the immense number of compounds based on the first order valences.
As indicated earlier, one component of any true chemical compound must have a negative displacement of four or less, as it is only through the establishment of an equilibrium between such a negative displacement and an appropriate positive displacement that the compound comes into existence. The elements with the required negative displacement are those which comprise Division IV, and it follows that every compound must include at least one Division IV element, or an element which has acquired Division IV status by valence enhancement. If there is only one such component, the positive-negative orientation is fixed, as the Division IV element is necessarily the negative component. Where both components are from Division IV, however, one normally negative element must reorient itself to act in a positive capacity, and a question arises as to which retains its negative status.
The answer to this question hinges on the relative negativity of the elements concerned. Obviously a small displacement is more negative than a large one, since it is farther away from the neutral point where positive and negative displacements of equal magnitude are equivalent. Within any one group the order of negativity is therefore the same as the displacement sequence. In Group 2B, for instance, the most negative element is chlorine, followed by sulfur, phosphorus, and silicon, in that order. This means that the negative component in any Division IV chlorine-sulfur combination is chlorine, and the product is a compound such as SCl2, not ClS or Cl2S. On the other hand, the compound P2S3 is in order, as phosphorus is normally positive to sulfur.
Where the electric displacements are equal, the element with the smaller magnetic displacement is the more negative, as the effect of a greater magnetic displacement is to dilute the negative electric rotation by distributing it over a larger total displacement. We therefore find ClF3 and IBr3, but not FCl3 or BrI3. The magnitude of the variation in negativity due to the difference in magnetic displacement is considerably less then that resulting from inequality of electric displacement, and the latter is therefore the controlling factor except where the electric displacements are the same in both components.
On the foregoing basis, all elements of Divisions I, II, and III are positive to Division IV elements. The displacement 4 elements on the borderline between Divisions III and IV belong to the higher division when combined with elements of lower displacement, and when elements lower in the negative series acquire valences of 4 or more through enhancement or reorientation they also assume Division III properties and become positive to the other Division IV elements. Thus chlorine, which is negative to oxygen in the purely Division IV compound OCl2, is the positive component in Cl2O7. Similarly, the normal relations of phosphorus and sulfur, as they exist in P2S3, are reversed in S3P4, where sulfur has the valence 4.
Hydrogen, like the displacement 4 members of the higher groups, is a borderline element, and because of its position is able to assume either positive or negative characteristics. It is therefore positive to all purely negative elements (Division IV below valence 4), but negative to all strictly positive elements (Divisions I and II), and to the elements of Division III. Because of its lower magnetic displacement, it is also negative to the higher borderline elements: carbon, silicon, etc. The fact that hydrogen is negative to carbon is particularly significant in view of the importance of the carbon-hydrogen combination in the organic compounds.
Another point that should be noted here is that when hydrogen acts in a positive capacity, it does so as a Division III element, not as a member of Division I. Its +1 valence is therefore magnetic. This is why hydrogen was assigned only to the negative position in the revised periodic table, rather than giving it two positions, as has been customary.
The variation in negativity with the size of the magnetic displacement has the effect of extending the Division III behavior into Division IV to a limited extent in the higher groups. Lead, for example, has practically no Division IV characteristics, and bismuth has less than its counterparts in the lower groups. At the lower end of the atomic series this situation is reversed, and the Division IV characteristics extend into Division III, as an alternative to the normal positive behavior of some of the elements of that division. Silicon, for instance, not only forms combinations such as MnSi and CoSi3, which, on the basis of the information currently available, appear to be intermetallic compounds similar to those of the higher Division III elements, but also combinations such as Mg2Si and CaSi2, which are probably true compounds analogous to Be2C and CaC2. Carbon carries this trend still farther and forms carbides with a wide variety of positive components.
In the 2A group, the Division IV characteristics extend to the fifth element, boron. This is the only case in which the fifth element of a series has Division IV properties, and the behavior of boron in compound formation is correspondingly unique. In its Division I capacity, as the positive component in compounds such as B203, boron is entirely normal. But its first order negative valence would be -5. Formation of compounds based on this -5 valence conflicts with the previously stated limitation of the negative valence to a maximum of four units. Boron therefore shifts to an enhanced negative valence, adding two positive units to its first order value of -5, with a resultant of -3. The direct combinations of boron with positive elements have such structures as FeB and Cu3 B2. However, many of the borides have complex structures in which the effective valences are not as clearly indicated. This raises a question as to whether boron may be an exception to the rule limiting the maximum negative valence to -4, and may utilize both the -5 and -3 valences. This issue will be considered in the next chapter.
