CHAPTER 21

Ring Compounds

The second major classification of the organic compounds is that of the ring compounds. These ring structures are again divided into three sub-classes. In two of these, the positive components of the magnetic neutral groups of the rings are carbon atoms: the cyclic, or alicyclic, compounds in which the predominant carbon valence is two, and the aromatic compounds in which this valence is one. In the third class, the heterocyclic compounds, one or more of the carbon atoms in the ring is replaced by an atom of some other element. All of these classes are further subdivided into mononuclear and polynuclear divisions, the basic structure of the latter being formed by a condensation or fusion of two or more rings. It should be understood that the classifications are not mutually exclusive. A compound may consist of a ring joined to one or more chains; a chain compound may have one paraffinic and one olefinic branch; a cyclic ring may be joined to an aromatic ring; and so on.

As in the chain compounds, a parallel classification divides the ring compounds into families characterized by the nature of the negative components: hydrocarbons, alcohols, amines, etc. The normal cyclic hydrocarbon, a cyclane, or cycloparaffin, is a simple ring of CH₂ neutral groups. The general formula can be expressed as -(CH₂)_n Beginning with cyclopropane (N=3) normal cyclanes have been prepared with all values of n up to more than 30. The neutral groups in these rings are identical with the CH₂ neutral groups in the chain compounds, and they may be expanded in the same manner by CH₂ additions. Corresponding to the branched chain compounds we therefore have branched rings such as ethylcyclohexane, -(CH₂)₅ (CH•CH₂•CH₃)-, and 1-methyl-2ethyl cyclopentane, -CHCH₃•(CH•CH₂•CH₃)•(CH₂)_3-.

In the notation used herein, the neutral groups will be clearly identified by parentheses or other means, and the positive-negative order will be preserved within these groups as in the neutral groups of the chain compounds. To identify the substance as a ring compound and to show that the end positions in the straight line formula have no such special significance as they do in the chain compounds, dashes will be used at each end of the ring formula as in the examples given. If two or more rings are present, or if a portion of the compound is outside the ring, the positions of the dashes will so indicate. While any group could be taken as the starting point in expressing the formula of a single ring, the order of the usual numbering system will be followed as far as possible, to minimize the deviations from familiar practice. The branch names such as 1-methyl-2-ethyl are then clearly indicated by the formula.

Replacement of all of the valence two groups in the cyclic ring by valence one groups, where such replacement is possible, converts the cyclic compound into an aromatic. In general, however, the distinctive aromatic characteristics do not appear unless the replacement is complete, and the intermediate structures in which CH or its equivalent has been substituted for CH₂ in only part of the ring positions will be included in the cyclic classification. Since the presence of the remaining CH₂ groups is the principal determinant of the molecular properties, the predominant carbon valence, in the sense in which that term is used in defining the classes of ring compounds, is two, even where there are more CH than CH₂ groups in the molecule.

As mentioned earlier, the probabilities favor association of like forces in the molecular compounds. The CH₂ groups have sufficient latitude in their geometric arrangement to be able to compensate for substantial variations, and single CH₂ groups can therefore fit into the molecular structure without difficulty, but the CH groups have very little geometric leeway, and for that reason they nearly always exist in pairs. This does not mean that the individual group is positively barred from existing separately, and in some of the more complex structures single CH groups can be found, but in the simple rings the pairs are so much more probable than the odd numbers of groups that the latter are excluded.

The first two-group substitution in the cyclanes produces the cyclenes, or cycloolefins. A typical compound is cyclohexene, -(CH₂)₄•(CH)₂ . The designations cycloparaffin and cycloolefin are not appropriate, in view of the findings of this work, as the cycloparaffins contain no carbon atoms with the characteristic paraffin valence, and it is the substitution of two acetylene valence groups into the CH₂ rings that forms the cycloolefins. The names cyclane and cyclene are therefore preferable.

Substitution of two more CH groups into the ring produces the cyclodienes. The existence of two CH•CH pairs in these compounds introduces a new factor in that the positions of the pairs within the ring may vary. No question of this kind arises in connection with cyclopentadiene, -(CH)₄•CH₂, the first compound in this series, but in cyclohexadiene two different arrangements are possible: -(CH)₄• (CH₂)₂ which is known as 1,3-cyclohexadiene, and -(CH)₂•CH₂•(CH)₂•CH₂ which is 1,4-cyclohexadiene.

Negative hydrogen atoms in the cyclic compounds may be replaced by equivalent atoms or groups in the same manner as those in the magnetic neutral groups of the chain compounds. The resulting products, such as cyclohexyl chloride, -(CH₂)₅•CHCI-, cyclohexanol, -(CH₂)₅•CHOH-, cyclohexylamine, -(CH₂)₅•CHNH₂, etc., have properties quite similar to those of the equivalent chain compounds: chlorides, alcohols, amines, and so on.

There are no atomic groups in the normal cyclic rings which have an amount of freedom of geometric arrangement comparable to that of the radicals at the two ends of the aliphatic chains, and the substituents which are limited to the radicals in the chains do not appear at all in the cyclic compounds unless a branch becomes long enough to put the end group beyond the range of the forces originating in the ring. In this case the structure is in effect a combination chain and ring compound. Because of this geometric restriction the range of substituents in the normal types of cyclic compounds is considerably narrower than in the chains. In addition to those already mentioned, Cl, OH, and NH₂, the primary list includes the remaining halogens, oxygen, CN, and CO•OH.

The compounds formed by direct substitution of oxygen for the two hydrogen atoms of the CH₂ group are named as ketones, but they do not have the ketone structure, as the resulting CO group is part of the ring and is a magnetic neutral group. One substitution produces cyclohexanone, -(CH₂)₅•CO-. A second results in a compound such as 1,3-cyclohexanedione,
-CO•CH₂•CO•(CH₂)₃-. The CO substitution can extend all the way to cyclohexane hexone, -(CO)₆, in which no hydrogen remains. It is also possible to make the oxygen substitution by means of a valence one combination instead of the full valence two replacement, in which case we obtain a compound such as cyclohexyl methyl ether, -(CH₂)₅•(CH•OCH₃)-.

Additional families of compounds are produced both by secondary substitutions, which result in structures on the order of cyclohexyl acetate, -(CH₂)₅•CH(O•CO•CH₃)-, and by parallel substitutions in two or more neutral groups. An example of the type of structure that is produced by the multiple substitutions is 1,2,3-cyclopropanetricarboxylic acid, -(CH•CO•OH)₃-. The naturally occurring compounds of this cyclic class are highly branched rings beginning with such substances as menthol, -CHCH₃•CH₂•CHOH•(CH•CHCH₃•CH₃)•(CH₂)₂, and extending to very complex structures, but they follow the same general structural patterns as the simpler cyclic compounds, and will not require additional discussion in the present connection.

As mentioned earlier, the CH₂ groups have a considerable degree of structural latitude because of their three-atom composition. The angle between the effective lines of force varies from about 120 degrees in cyclopropane to less than 15 degrees in the largest cyclic rings thus far studied. The two-atom groups such as CH do not have this structural freedom, and are restricted to a narrow range in the vicinity of 60 degrees. The theoretically exact limits have not yet been determined, but the difficulties involved in the preparation of derivatives of cyclooctatetraene, -(CH)₈, indicate that this compound is at the extreme limit of stability. This would suggest a maximum deviation of about 15 degrees from the 60 degree angle of the six-member ring. The atoms of which the molecular compounds are composed have a limited range in which they can assume positions above or below the central plane of the molecule. The actual angles between the effective lines of force will therefore deviate slightly from the figures given above, which are based on positions in the central plane, but this does not affect the point which is being made, which is that the cyclic ring is very flexible, whereas the aromatic ring is practically rigid.

As long as there is even one CH₂ group in the ring it has the cyclic flexibility. Cyclopentadiene can exist in spite of the rigidity of the portion of the ring occupied by the four CH groups because the CH₂ group that completes the structure is able to accommodate itself to the position necessary for closing the ring. But when all of the three-atom groups have been replaced by two-atom groups or single atoms the ring assumes the aromatic rigidity. Cyclobutadiene, for example, would consist of four CH groups only, and the maximum deviation of the CH lines of force, somewhere in the neighborhood of 75 degrees, is far short of the 90 degrees that would be required for closure of the cyclobutadiene ring. All attempts to produce such a compound have therefore failed.

The properties of the various ring compounds are dependent to a considerable degree on this question as to whether the members of the rings are restricted to certain definite positions, or have a substantial range of variability within which they can adjust to the requirements for combination. In view of this natural line of demarcation, the aromatic classification, as used in this work, is limited to the rigid structures, specifically to those compounds composed entirely of valence one CH groups or their monovalent substitution products, except for such connecting carbon atoms as may be present.

Because of the limitations on the atomic positions, the aromatic compounds, with the exception of cyclooctatetraene, are confined to the six-member rings, the valence one equivalents of cyclohexane and its derivatives, and there are no aromatic analogs of cyclobutane, cycloheptane, etc. The structural rigidity therefore limits the compound forming versatility of the aromatic rings to a substantial degree, but this is more than offset by other effects of the same factor. The locations in the chain compounds which are open to the greatest variety of combinations are the ends of the chain and its longer branches, if any. In the aromatic rings every ring location has, to some degree, the properties of an end. Also, because of the rigidity of the ring, the maximum intergroup distance 1-3 in the ring is about ten percent less than the distance between the equivalent groups in the aliphatic chain, after making an allowance for the small amount of flexibility that does exist. This brings some additional combinations of elements within the limit of effectiveness of the free electric displacements, and in these rings we find not only groups such as COH, CCI, CNH₂, etc., which are the valence one equivalents of the combinations that make up the cyclic rings and the interior portions of the chain compounds, but also other combinations such as CNO₂ and CSH which are just beyond the magnetic neutral limits in the non-aromatic structures. The number of available combinations in which the neutral group CO accompanies the negative radical is similarly increased.

Secondary substitutions extend the length and diversity of the magnetic neutral groups of the ring, and produce a wide variety of single branch compounds on the order of isobutyl benzene, -(CH)₅•(C•CH₂•CHCH₃•CH₃)- and N-ethyl aniline, -(CH)₅•(C•NH•CH₂•CH₃)-, but the principal field for variability in the mononuclear aromatics lies in their capability of multiple branching. The aromatic rings not only have a greater variety of available substituents than any other type of molecular compound, but also a larger number of locations where these substituents may be introduced. This versatility is compounded by the fact that in the rings, as in the chains, the order of sequence of the groups has a definite effect on the properties of the compound. The behavior of 1,2-dichlorobenzene, -(CCl)₂•(CH)₄, for instance, is in many respects quite different from that of 1,4-dichlorobenzene, -CCl•(CH)₂•CCl•(CH)₂ .

A significant feature of the aromatic rings is their ability to utilize larger numbers of the less versatile substituents. For example, the limitation of such groups as NO₂ to the negative radical in the chains means that only one such group can exist in any chain compound, unless a branch becomes so long that the compound is in effect a union of two chains. In the aromatic ring this limitation is removed, and compounds with three or four of the highly reactive nitro groups in the six-member ring are common. The list includes such well-known substances as picric acid (2,4,6-trinitrophenol), -COH•CNO₂•CH•CNO₂•CH•CNO₂-, and TNT (2,4,6-trinitrotoluene), -CH₃•CNO₂•CH•CNO₂•CH•CNO₂- .

Since there is only one hydrogen atom in the CH group, the direct substitutions in the aromatic rings are limited to valence one negative components. In order to establish a valence equilibrium with a bivalent atom or radical two of the aromatic rings are required. These bivalent atoms or groups therefore constitute a means whereby two rings can be joined. Diphenyl ether, for example, has the structure -(CH)₅•C-OC•(CH)₅-, in which the oxygen atom is not a member of either ring but participates in the valence equilibrium. The bivalent negative radical NH similarly produces diphenylamine, -(CH)₅•C-NH-C•(CH)₅-.

Each of these rings is a very stable structure with a minimum of eleven constituent atoms, and a possibility of considerable enlargement by substitution. This method of joining rings is therefore a readily available process whereby stable molecules of large size may be constructed. Further additions and substitutions may be made not only in the rings and their branches, but in the connecting link as well. Thus the addition of two CH₂ groups to diphenyl ether produces dibenzyl ether, -(CH)₅•CCH₂•O•CH₂ C•(CH)₅-.

According to the definition of an aromatic compound, these multiple ring structures are not purely aromatic, as the connecting links do not qualify. This is a situation which we will encounter regardless of the manner in which the various organic classifications are set up, as the more complex compounds are primarily combinations of the different basic types of structure. Ordinarily a compound is classified as a ring structure if it contains a ring of any kind, even though the ring may be only a minor appendage on a long chain, and it is considered as an aromatic if there is at least one aromatic ring present.

In the multiple ring compounds the combination (CH)₅•C, which is a benzene ring less one hydrogen atom, acts as a monovalent positive radical, the phenyl radical, and the simple substituted compounds can be named either as derivatives of benzene or as phenyl compounds; i.e., chlorobenzene or phenyl chloride. The net positive valence one is the valence condition in which the ring is left when a hydrogen atom is removed, but this net valence is due entirely to the +1 valence of the lone carbon atom from which the hydrogen atom was detached, all other groups being neutral, and it does not necessarily follow that the carbon valence will remain at +1. As emphasized earlier, valence is simply a matter of rotational orientation, and when acting alone any atom can assume any one of its possible valences, providing that there are no specific obstacles in the environment. The lone carbon atom is therefore free to accommodate itself to different environments by reorientation on the basis of any of its alternate valences: +2, +4, or -4.

If two phenyl radicals are brought together, the inter-atomic forces will tend to establish an equilibrium. A valence balance is a prerequisite for a force equilibrium, and the carbon atoms will therefore reorient themselves to balance the valences. There are two possible ways of accomplishing this result. Since carbon has only one negative valence, -4, one carbon atom takes this valence, and a second must assume the +4 valence in order to arrive at an equilibrium. In a direct combination of two phenyl groups these valence changes can be made in the two independent carbon atoms, without modifying the neutral groups in any way, and this is therefore the most probable structure in such compounds as biphenyl, -(CH)₅•C-C•(CH)₅-. A similar balanced pair of positive and negative valence 3 nitrogen atoms may be introduced, in combination with the valence 4 carbon atoms, to form azobenzene, -(CH)₅•CNNC•(CH)₅-.

The alternative is to make both valence changes in the same phenyl group, giving the lone carbon atom the -4 valence and increasing the v,alence of the carbon atom in an adjacent neutral group from +1 to +4. The product is a ring in which there are four CH neutral groups, a CH group with a net valence of +3, and a single carbon atom with the -4 valence. By this means the phenyl group is changed from a univalent positive radical, C•(CH)₅, to a univalent negative radical, (CH)₄•CH•C. Like the methyl group, which can act either as a positive radical CH₃ with valence +1, or as a negative radical CH₂•H with valence -1, the phenyl group is able to combine with substances of either valence type, taking the negative valence in combination with a positive component, and the positive valence when combining with a negative atom or group. It is negative in all of the phenyl compounds of the metal-organic class, and not only forms compounds such as phenyl copper, Cu-C•(CH)₅-, and diphenyl zinc, Zn(-C•(CH)₅-)₂, but also combination phenyl-halide structures like phenyl tin trichloride, SnCl₃-C•(CH)₅-.

In combination with the CH₃ radical the phenyl group is positive. Either radical can take either valence, but the methyl group probabilities are nearly equal, while the positive valence is more probable in the phenyl group, since it involves no change in the benzene ring other than the removal of a hydrogen atom. The combination -(CH)₅•CCH₃- is therefore toluene, with positive phenyl and negative methyl (carbon valence two), rather than phenyl methane, which would have negative phenyl and positive methyl (carbon valence four).

This option is not available in combination with other hydrocarbon radicals, or with carbon itself, and in such compounds the phenyl radical replaces hydrogen, and is negative. An additional phenyl substitution in toluene, for example, reduces the CH₃ radical to CH₂. This group cannot have the -2 net valence that would be necessary for combination with positive phenyl radicals, and both of the phenyl groups assume the negative status in the resulting compound, diphenyl methane. The olefinic and acetylenic benzenes likewise have this type of structure in which the phenyl radical is negative. Styrene, for instance, is not vinyl benzene, -(CH)₅•C-CH₂•CH, as that combination would contain two positive components and no negative. It is phenyl ethylene, CH•CH₂•-C•(CH)₅-, in which CH is positive and the phenyl group is negative.

An interesting phenyl compound is phenyl acetylene, the conventional formula for which is C₆HS•C•CH. On the basis of our finding that hydrogen is negative to carbon, the hydrogen atom in the acetylene CH would have to be negative. But this is not true, as it can be replaced by sodium. It seems evident, then, that this is phenyl carbide, -(CH)₅•CC₂H, a compound similar to butadiyne, which we have already identified as a carbide, C•CH•C₂H. As noted previously, the relative negativity of carbon and hydrogen has no meaning with reference to the carbide radical, which has a net negative valence, and cannot be other than negative regardless of what element or group it combines with. According to the textbooks, the phenyl compound is identified as an acetylene because “it undergoes the typical acetylene reactions.” But so does any other carbide. The acetylene lamp was a “carbide” lamp to the cyclists of an earlier day.

Like the phenyl radical, the cyclic radicals can accommodate themselves to either the positive or negative position in the molecule. These radicals, too, are positive in the monosubstituted compounds. A methyl substitution produces hexahydro toluene, not cyclohexyl methane. But if there are two cyclic substitutions in a methyl group they are both negative, and dicyclohexyl methane is a reality.

At this point it will be desirable to examine the effects of the various modifications of the ring structure on the cohesion of the molecule. We may take the ben_zene ring as the basic aromatic structure. Textbooks and monographs on the aromatic compounds typically contain a chapter, or at least a lengthy section, on the “benzene problem.”⁶⁹ The problem, in essence, is that all of the evidence derived from observation and experiment indicates that the inter-atomic forces and distances between any two of the six CH groups in the ring are identical, but no theory of the chemical “bond” has been able to account for the structure of the benzene molecule without utilizing two or more different kinds of bonds. The currently favored “solution” of the problem is to sweep it under the rug by postulating that the structure alternates, or “resonates,” between the different bond arrangements.

The development of the Reciprocal System of theory now shows that the forces between the groups in the benzene ring are, in fact, identical. As has been emphasized throughout the preceding discussion, however, the existence and nature of chemical compounds is not determined by the cohesive forces between the atoms of the different elements, but by the directional relationships which the atomic rotations must assume in order to permit elements with electric rotation in time to establish stable force equilibria in space. The findings of this theoretical development agree that the orienting effects which enable CH groups to combine into the benzene ring are of two different types, a short range effect and a long range effect, but they also reveal that the nature of the orienting influences has no bearing on the magnitude of the inter-atomic forces, and this explains why no difference in these forces can be detected experimentally. The forces between any two of the CH neutral groups in the ring are identical.

Inasmuch as the orienting factors cause the atoms to align their rotations in certain specific relative directions, they are, in a sense, forces, but in order to distinguish them from the actual cohesive forces that hold the atoms, groups, and molecules together in the positions determined by these orienting factors we are using the term “effects” rather than “forces” in application to the orientation, even though this introduces an element of awkwardness into the presentation. The nature of these effects, as they apply to the benzene ring, can be illustrated by an orientation diagram of the kind previously introduced.

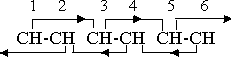
The pairs of CH groups, 1-2, 3-4, and 5-6, in the diagram, are held in the combining positions by the orienting effects of a directional character that are exerted by all magnetic groups or compounds. Alternate groups, 1-3, 2-4, etc., are within unit distance, and therefore within the effective range of these orienting effects. The primary effect of group l, for instance, is directed toward group 2, but group 3 is also within unit distance, and consequently there is a long range 1-3 secondary effect as well as a short range 1-2 primary effect. Because of the directional nature of these orienting effects there is no 2-3 primary effect, but the pairs 1-2 and 3-4 are held in position by the 1-3 and 4-2 secondary effects.

If we replace one of the hydrogen atoms with some negative substituent, the orientation situation is unchanged. The new neutral group, or that portion of it which is within the range of the ring forces if the group is a long one, takes over the functions of the CH group without alteration. However, removal of a hydrogen atom and conversion of the benzene molecule into a positive phenyl radical changes the orientation pattern to

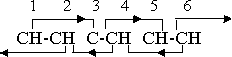
The secondary effect 3-5 has now been eliminated, as the lone carbon atom does not have the free electric rotation characteristic of the magnetic groups or compounds, but the remaining orientation effects are still adequate to hold the structure together. The further valence change that is necessary if the phenyl radical is to assume a negative valence similarly eliminates the 4-2 secondary effect, as group 4 is no longer magnetic. However, the two carbon atoms and one hydrogen atom combine into a radical CCH, with a net valence of -1. This radical has no orienting effect on its neighbors, but the adjoining magnetic neutral groups do exert their effect on it. The orientation pattern is

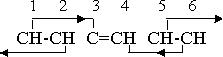
As previously explained, the carbon atoms in the CCH combination have valences +4 and -4. If we remove the hydrogen atom from this group we obtain a ring in which four CH neutral groups are combined with two individual carbon atoms. This structure is neutral and is capable of existing as an independent compound, but, like the methylene molecule, it does not actually do so, because it has a strong tendency to form a double ring. The four CH groups which are attached to the C-C combination can be duplicated on the opposite side of the C-C line of action, forming another similar ring which utilizes the same pair of carbon atoms as part of its ring structure. The fact that the effects originating from the free electric rotations are exerted on the carbon atoms by the CH groups on one side does not in any way interfere with the existence of similar effects on the other side. The orientation relations in the second ring are identical with those of the first. Neither ring can now recapture a hydrogen atom and become a phenyl radical because the presence of the other ring prevents the approach of the free hydrogen atoms. The double ring compound therefore has a high degree of stability.

This compound is naphthalene, -(CH)₄•C=C•(CH)₄, a condensed ring aromatic hydrocarbon. When used in the formula of a compound in this work, the double mark between two carbon atoms is a symbol indicating the condensed ring type of structure in which the rings are joined at two positions rather than at a single position as in compounds such as biphenyl. It has no implications of the kind associated with the “double bonds” of the electronic theory.

A third ring added in the same manner produces anthracene. Further similar additions in line result in a series of compounds: naphthacene, pentacene, and so on. But it is not necessary that the additions be made in line, and each of these compounds is accompanied by others which have the same composition, but different structures. For instance, the four ring compounds of the naphthacene composition, C₁₈H₁₂, include chrysene, naphthanthracene, 3,4-benzophenanthrene, and triphenylene. Pyrene has the same four rings, but a more compact structure, and a composition C₁₆H₁₀.

The structural behavior of the condensed rings is essentially the same as that of the single benzene rings. They join to form compounds such as binaphthyl and bianthryl; they act as radicals (naphthyl, anthryl, phenanthryl, etc.); they attach more rings by substitution for hydrogen to produce compounds such as triphenyl anthracene; and they form a great variety of compounds by utilizing the other negative substituents available to the aromatic rings. Many interesting and important compounds are included in this category, but no new structural features are involved, and they are therefore outside the scope of the present discussion.

The two CH groups of the middle ring of the anthracene structure are not necessary for stability, and they can be eliminated. The resulting compound is biphenylene, -(CH)₄•CC=CC•(CH)₄ . A structure with only one CH group in the middle ring, intermediate between anthracene and biphenylene, is ruled out by the low probability of the continued existence of a single CH group, but a similar compound can be formed by putting a CH₂ group in the intermediate position, as the CH₂ groups are not restricted to pairs. The new compound is fluorene. Another CH₂ group in the opposite position restores the anthracene structure with a cyclic middle ring. This compound is dihydroanthracene.

As previously mentioned, a ring with even one CH₂ group deviates substantially from the typical aromatic behavior, and any such ring is classified with the cyclic structures, but this effect is confined to the specific ring, and any adjacent aromatic rings retain their aromatic character. Such compounds as fluorene and dihydroanthracene should therefore be regarded as combination cyclic-aromatic structures. These compounds occur in large numbers and in great variety, but the principles of combination are the same as in the purely aromatic compounds, and do not need to be repeated. Since the cyclic compounds are less stable than the corresponding aromatics, the combination structures do not cover as large a field as the aromatic compounds, but a very stable structure such as that of naphthalene does extend through the entire substitution range. Beginning with the purely aromatic compound, successive pairs of hydrogen atoms can be added all the way to the purely cyclic compound, decahydronaphthalene.

The reduction in the variety of combination structures due to the fact that the cohesive force in the cyclic ring is weaker than that in the aromatic ring is offset to some extent by the ability of the CH₂ groups to form rings of various sizes. 1,2,3,4-tetrahydronaphthalene, for instance, can drop one of its CH₂ groups, forming indane, -(CH)₄•C=C•(CH₂)₃-. Because of the CH₂ flexibility, the cyclic ring in this compound is still able to close even if two of the remaining CH₂ groups are replaced by CH. This produces indene, -(CH)₄•C=C•(CH)₂•CH₂ .

Polynuclear cyclic compounds are formed in the same manner as the polynuclear aromatic and combination structures, but not in as great a number or variety. Corresponding to biphenyl and its substitution products are dicyclopentyl, dicyclohexyl, etc., and their derivatives; triphenyl methane has a cyclic equivalent in tricyclohexyl methane; the cyclic analog of naphthalene is bicyclodecane, and so on.

The last major division of the ring compounds is the heterocyclic class, in which are placed all compounds in which any of the carbon atoms in the cyclic or aromatic rings are replaced by other elements. The principal reason for setting up a special classification for these compounds is that most of the substitutions of other elements for carbon require valence changes of one kind or another, unlike the substitutions for hydrogen, which normally involve no valence modifications, except in those cases where two valence one hydrogen atoms are replaced by one valence two substituent.

Some of the heterocyclic substitutions are of this two for one character, and in those cases the normal cyclic or aromatic structure is not altered. For example, if we begin with quinone,
-(CH)₂•CO•(CH)₂•CO-, an aromatic carbon compound, and replace two of the CH groups with NH neutral groups we obtain uracil, -NH•CO•NH•CH•CH•CO-. One more similar pair replacement removes the last of the hydrocarbon groups and results in urazine, -NH•CO•NH•NH•CO•NH-. In the compound cyclohexane hexone previously mentioned all of the hydrogen has been replaced, and in borazole, -BH•NH•BH•NH•BH•NH-, all carbon is eliminated. All of these heterocyclic compounds are composed entirely of two-member magnetic neutral groups, and therefore have the benzene structure: six groups arranged in a rigid aromatic ring.

More commonly, however, the heterocyclic substituent is a single atom or a radical, and such a substitution requires a valence change in some other part of the ring to maintain the valence equilibrium. Substitutions therefore often take place in balanced pairs. In pyrone, -(CH)₂•CO•(CH)₂•O-, for example, the CO combination is not a neutral group, but a radical with valence +2 which balances the -2 valence of the oxygen atom. The CH₂ radical, in which carbon also has its normal valence +4, has the same function in pyran, -(CH)₂•CH₂•(CH)₂•O-. Substitution of two nitrogen atoms with the balanced valences of +3 and -3 in the aromatic ring produces a diazine. If the nitrogen atoms are in the 1,2 positions the compound is pyridazine, -N•N•(CH)₄ . The properties of the 1,3 and 1,4 compounds are enough different from those of pyridazine that they have been given distinctive names, pyrimidine and pyrazine, respectively.

Since the positive and negative radicals in a ring have no fixed positions similar to the two ends of the chains, it is not possible to indicate their status by their positions as we do in the formulas we are using for the chain compounds. Some appropriate method of identification probably should be devised in order to make the formula as representative of the actual structure as possible, but this is not necessary for the purposes of the present work, and can be left for later consideration.

The following orientation diagrams for pyrone and pyridazine are typical of those for heterocyclic compounds with single atom or radical substitutions:

If the valence equilibrium is not achieved in this manner by means of a pair of substitutions, a valence change in one of the neutral groups is necessary. A single nitrogen atom substituted into the ring requires a +3 valence elsewhere in the structure to counterbalance the negative nitrogen valence. This is readily accomplished by a shift of one of the carbon valences to +4. The reconstructed ring then consists of a nitrogen atom, valence -3, a CH radical, valence +3, and four CH neutral groups. This compound is pyridine, -(CH)₅•N-. Hydrogenation can be carried out by steps through intermediate compounds all the way to the corresponding cyclic structure, piperidine, -(CH₂)₅•NH-.

When oxygen, or another valence two negative component, is introduced into the aromatic ring the necessary valence balance may be attained by a simultaneous replacement of one of the CH neutral groups by a CH₂ radical, as already noted in the case of pyran. Or the required balance can be achieved without introduction of additional hydrogen if the carbon valences in two of the CH groups are stepped up to the +2 level (the primary magnetic valence), forming two CH radicals, each with valence +1. This leaves an unstable odd number of CH neutral groups in the six-member ring, but there is sufficient flexibility in the structure to enable a ring closure on a five-member basis, and stability is restored by ejecting a neutral group. The resulting compound is furan, -(CH)₄•O-, a five-member ring with one oxygen atom, two CH neutral groups, and two CH valence one positive radicals. Substituting sulfur instead of oxygen produces thiophene, -(CH)₄•S-, while inserting the negative radical NH into the same position produces pyrrole, -(CH)₄•NH-. Each of these furan type compounds also exists in the cyclic dihydro and tetrahydro forms. The furan orientation pattern is

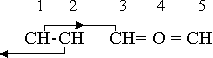
The essential feature of all of these five-member rings of the furan class is a valence equilibrium in which three of the five components participate, the two remaining components being the neutral groups that furnish the ring-forming capability. In furan the equilibrium combination is C⁺-O•2-C⁺. Formation of a similar combination with nitrogen in the negative position requires that some element or radical positive to nitrogen take the positive position, and in the heterocyclic division nitrogen itself commonly accepts this role. The most probable valence under these conditions is +3, as in hydrazine. The two nitrogen valences, +3 and -3, are then in equilibrium, and in this case the fifth component of the five-member ring must be a neutral group. Since it is a single group, it is the cyclic group CH₂, and the neutral trio is N⁺³-N^-3-CH₂⁰. The compound is isopyrazole, -N•CH•CH•CH₂•N-. An alternate group arrangement produces isoimidazole, -N•CH₂•N•CH•CH-. A variation of this structure moves a hydrogen atom from the CH₂ group to the positive nitrogen, which changes the neutral combination to NH ⁺²-N^-3-CH⁺¹. The compounds formed on this basis are pyrazole, -N•(CH)₃•NH-, and imidazole, -N•CH•NH•CH•CH-.

From these basic heterocyclic types a great variety of condensed systems such as coumarone (benzofuran), indole (benzopyrrole), quinoline (benzopyridine), etc., can be formed by combination with other rings. Both the single rings and the condensed systems are then open to further enlargement by all of the processes of addition and substitution previously discussed, and a very substantial proportion of the known organic compounds belong to this class. From a structural standpoint, however, the basic principles involved in the formation of all of these compounds are those that have been covered in the preceding discussion.

In the foregoing pages we have encountered several kinds of isomerism, the existence of different compounds with the same composition. Some, such as the cyanides and isocyanides, differ only in valence; some, such as the straight chain and branched paraffins, differ in the position of the neutral groups; and some, such as the aldehydes and the ketones, differ in the assignment of the atoms of the constituent elements to the structural groups. Most of these isomers that we have examined thus far are distinct stable compounds. There are also some isomeric systems in which the two forms of a substance convert so readily from one to the other that they establish an equilibrium which varies in accordance with the conditions to which the compound is subject. This form of isomerism is known as tautomerism.

One of the familiar examples of tautomerism is that between the, “keto” and “enol” forms of certain substances. Ethyl acetoacetate COCH₃•CH₂•CO•(O•CH₂•CH₃), is the keto form of a compound that also exists in the enol form as the ethyl ester of hydroxycrotonic acid, COH•CHCH₃•CO•(O•CH₂•CH₃). The compound freely changes from one form to the other to meet changing physical and chemical conditions. This is another example of counterbalancing carbon and hydrogen valence changes, and it is an indication of the ease with which such changes can be made. In the radical COCH₃ the carbon valence is +4, and all hydrogen is negative. The transition to the enol form involves a drop in the carbon valence to +2, and one hydrogen atom shifts from -1 to +1 to maintain the balance. The CH₂ group in the radical is then superfluous, and it moves to the adjacent neutral group. The remainder of the molecule is unchanged.

The development of the Reciprocal System of theory has not yet been extended to a study of tautomerism. Nor has it been applied to those kinds of isomerism which depend on the geometrical arrangement of the component parts of the molecules, such as optical isomerism. These aspects of the general subject of molecular structure will therefore have to be left for later treatment.

This chapter is the last of the four that have been devoted to an examination of the structure of chemical compounds. In closing the discussion it will be appropriate to point out just how the presentation in these chapters fits into the general plan of the work, as defined in Chapter 2. The usual discussion of molecular structure, as we find it in the textbooks, starts with the empirical observation that certain chemical compounds-sodium chloride, benzene, water, ethyl alcohol, etc.-exist, and have certain properties, including different molecular structures. The theoretical treatment then attempts to devise plausible explanations for the existence of these observed compounds, their structures, and other properties. This present work, on the other hand, is entirely deductive. By developing the necessary consequences of the fundamental postulates of the Reciprocal System we find that in a universe of motion matter must exist; it must exist in the form of a series of elements; and those elements must have the capability of combining in certain specific ways to form chemical compounds. In this and the preceding chapters, the most important of the possible types of molecular structures have been derived from theory, and specific compounds have been characterized by composition and structure.

The second objective of the work is to identify these theoretical combinations with the observed chemical compounds. For example, we deduce purely from theory that there must exist a compound in the form of a chain of three groups of atoms, in which the first group contains three atoms of element number one and one atom of element number six, and has a net group combining power, or valence, of +1. The second group has two atoms of element number one and one of number six, and is neutral; that is, its net valence is zero. The third group has one atom of element number one and one of element number eight, and a valence of -1. This theoretical composition and structure are in full agreement with the composition of the observed compound known as ethyl alcohol, and with the structure of that compound as deduced from physical and chemical observation and measurement. We are thus entitled to conclude that ethyl alcohol is the chemical compound existing in the physical universe that corresponds to the compound which must exist in the theoretical universe of the Reciprocal System. In other words, we have identified the theoretical compound as ethyl alcohol.

The great majority of the identifications cited in the preceding pages are unequivocal-almost self-evident, we may say-and this agreement establishes the validity of both the theoretical development and the empirical determination of the molecular structures. Where there are discrepancies, some of them, such as the one involved in the structure of ethylene, are quite easily explained. However, as the size and complexity of the molecules increases, the number and variety of the possible modifications of the theoretical structure also increases, in even greater proportion, and the observable differences between the various modifications decrease. The validity of the identifications is therefore less certain than in the case of the smaller and simpler molecules, but this does not mean that there is any additional uncertainty with respect to the existence of the more complex theoretical compounds. It merely means that the available empirical information is not adequate to permit a definite decision as to which of the observed compounds corresponds to a particular theoretical structure. It can be expected, therefore, that further investigation will clear up most of these questions.

The discussion of chemical compounds in this and the preceding three chapters completes the description of the primary physical entities, the actors in the drama of the physical universe. In the next volume we will begin applying the theoretical findings to an examination of the drama itself: the action in which these entities are involved.