Chapter 5
Heat
If an atom is subjected to an external force of a transient nature, such as that involved in a violent contact a motion is imparted to it. Where the magnitude of the force is great enough the atom is ejected from the time region and the inter-atomic equilibrium is destroyed. If the force is not sufficient to, accomplish this ejection, the motion is turned back at some intermediate point and it becomes a vibratory, or oscillating, motion.
Where two or more atoms are combined into a molecule, the molecule becomes the thermal unit. The statements about atoms in the preceding paragraph are equally applicable to these molecular units. In order to avoid continual repetition of the expression “atoms and molecules,” the references to thermal units in the discussion that follows will be expressed in terms of molecules, except where we are dealing specifically with substances such as aggregates of metallic elements, in which the thermal units are definitely single atoms. Otherwise the individual atoms will be regarded, for purposes of the discussion, as monatomic molecules.
The thermal motion is something quite different from the familiar vibratory motions of our ordinary experience. In these vibrations that we encounter in everyday life, there is a continuous shift from kinetic to potential energy, and vice versa, which results in a periodic reversal of the direction of motion. In such a motion the point of equilibrium is fixed, and is independent of the amplitude of the vibration. In the thermal situation, however, any motion that is inward in the context of the fixed reference system is coincident with the progression of the natural reference system, and it therefore has no physical effect. Motion in the outward direction is physically effective. From the physical standpoint, therefore, the thermal motion is a net outward motion that adds to the gravitational motion (which is outward in the time region) and displaces the equilibrium point in the outward direction.
In order to act in the manner described, coinciding with the progression of the natural reference system during the inward phase of the thermal cycle and acting in conjunction with gravitation in the outward phase, the thermal vibration must be a scalar motion. Here again, as in the case of the vibratory motion of the photons, the only available motion form is simple harmonic motion. The thermal oscillation is identical with the oscillation of the photon except that its direction is collinear with the progression of the natural reference system rather than perpendicular to it. However, the suppression of the physical effects of the vibration during the half of the cycle in which the thermal motion is coincident with the reference system progression gives this motion the physical characteristics of an intermittent unidirectional motion, rather than those of an ordinary vibration. Since the motion is outward during half of the total cycle, each natural unit of thermal vibration has a net effective magnitude of one half unit.
Inasmuch as the thermal motion is a property of the individual molecule, not an aspect of a relation between molecules, the factors that come into play at distances less than unity do not apply here, and the direction of the thermal motion, in the context of a stationary reference system is always outward. As indicated earlier, therefore, continued increase in the magnitude of the thermal motion eventually results in destruction of the inter-atomic force equilibrium and ejection of the molecule from the time region. It should be noted, however, that the gravitational motion does not contribute to this result, as it changes direction at the unit boundary. The escape cannot be accomplished until the magnitude of the thermal motion is adequate to achieve this result unassisted.
When a molecule acquires a thermal motion it immediately begins transferring this motion to its surroundings by means of one or more of several processes that will be considered in detail at appropriate points later in this and the subsequent volumes. Coincident with this outflow there is an inflow of thermal motion from the environment, and, in the absence of an externally maintained unbalance, an equilibrium is ultimately re ached at a point where inflow and outflow are equal. Any two molecules or aggregates that have established such an equilibrium with each other are said to be at the same temperature.
In the universe of motion defined by the postulates of the Reciprocal System, speed and energy have equal standing from the viewpoint of the universe as a whole. But on the low speed side of the neutral axis, where all material phenomena are located, energy is the quantity that exceeds unity. Equality of motion in the material sector is therefore synonymous with equal energy. Thus a temperature equilibrium is a condition in which inflow and outflow of energy are equal. Where the thermal energy of a molecule is fully effective in transfer on contact with other units of matter, its temperature is directly proportional to its total thermal energy content. Under these conditions,
|
E = kT |
(5-1) |
In natural units the numerical coefficient k is eliminated, and the equation becomes:
|
E = T |
(5-2) |
Combining Equation 5-2 with Equation 4-3 we obtain the general gas equation, PV = T, or in conventional units, where R is the gas constant.
|
PV = RT |
(5-3) |
These are the relations that prevail in the “ideal gas state.” Elsewhere the relation between temperature and energy depends on the characteristics of the transmission process. Radiation originates three-dimensionally in the time region, and makes contact one-dimensionally in the outside region. It is thus four-dimensional, while temperature is only one-dimensional. We thus find that the energy of radiation is proportional to the fourth power of the temperature.
|
Erad = kT4 |
(5-4) |
This relation is confirmed observationally. The thermal motion originating inside unit distance is likewise four-dimensional in the energy transmission process. However, this motion is not transmitted directly into the outside region in the manner of radiation. The transmission is a contact process, and is subject to the general inter-regional relation previously explained. Instead of E = kT4, as in radiation, the thermal motion is E2 = k’T4, or:
|
E = kT2 |
(5-5) |
A modification of this relation results from the distribution of the thermal motion over three dimensions of time, while the effective component in thermal interchange is only one-dimensional. This is immaterial as long as the thermal motion is confined to a single rotational unit, but the effective component of the thermal motion of magnetic rotational displacement n is only 1/n3 of the total. We may therefore generalize equation 5-5 by applying this factor. Substituting the usual term heat (symbol H) for the time region thermal energy E, we then have:
|
H = T2/n3 |
(5-6) |
The general treatment of heat in conventional physical theory is empirically based, and is not significantly affected by the new theoretical development. It will not be necessary, therefore, to give this subject matter any attention in this present work, where we are following a policy of not duplicating information that is available elsewhere, except to the extent that reference to such information is required in order to avoid gaps in the theoretical development. The thermal characteristics of individual substances, on the other hand, have not been thoroughly investigated. Since they are of considerable importance, both from the standpoint of practical application and because of the light that they can shed on fundamental physical relationships, it is appropriate to include some discussion of the status of these items in the universe of motion. One of the most distinctive thermal properties of matter is the specific heat, the heat increment required to produce a specific increase in temperature. This can be obtained by differentiating equation 5-6.
|
dH/dT = 2T/n3 |
(5-7) |
Inasmuch as heat is merely one form of energy it has the same natural unit as energy in general, 1.4918×10-3 ergs. However, it is more commonly measured in terms of a special heat energy unit, and for present purposes the natural unit of heat will be expressed as 3.5636×10-11 gram-calories, the equivalent of the general energy unit.
Strictly speaking, the quantity to which equation 5-7 applies is the specific heat at zero pressure, but the pressures of ordinary experience are very low on a scale where unit pressure is over fifteen million atmospheres, and the question as to whether the equation holds good at all pressures, an issue that has not yet been investigated theoretically, is of no immediate concern. We can take the equation as being applicable under any condition of constant pressure that will be encountered in practice.
The natural unit of specific heat is one natural unit of heat per natural unit of temperature. The magnitude of this unit can be computed in terms of previously established quantities, but the result cannot be expressed in terms of conventional units because the conventional temperature scales are based on the properties of water. The scales in common use for scientific purposes are the Celsius or Centigrade, which takes the ice point as zero, and the Kelvin, which employs the same units but measures from absolute zero. All temperatures stated in this work are absolute temperatures, and they will therefore be stated in terms of the Kelvin scale. For uniformity, the Kelvin notation (°K, or simply K) will also be applied to temperature differences instead of the customary Celsius notation (°C).
In order to establish the relation of the Kelvin scale to the natural system, it will be necessary to use the actual measured value of some physical quantity, involving temperature, just as we have previously used the Rydberg frequency, the speed of light, and Avogadro’s number to establish the relations between the natural and conventional units of time, space, and mass. The most convenient empirical quantity for this purpose is the gas constant. It will be apparent from the facts developed in the discussion of the gaseous state in a subsequent volume of this series that the gas constant is the equivalent of two-thirds of a natural unit of specific heat. We may therefore take the measured value of this constant, 1.9869 calories, or 8.31696×107 ergs, per gram mole per degree Kelvin, as the basis for conversion from conventional to natural units. This quantity is commonly represented by the symbol R, and this symbol will be employed in the conventional manner in the following pages. It should be kept in mind that R = 2/3 natural unit. For general purposes the specific heat will be expressed in terms of calories per gram mole per degree Kelvin in order to enable making direct comparisons with empirical data compiled on this basis, but it would be rather awkward to specify these units in every instance, and for convenience only the numerical values will be given. The foregoing units should be understood.
Dividing the gas constant by Avogadro’s number, 6.02486 x 1023 per g-mole, we obtain the Boltzmann constant, the corresponding value on a single molecule basis: 1.38044×10-16 ergs/deg. As indicated earlier, this is two-thirds of the natural unit, and the natural unit of specific heat is therefore 2.07066×10-16 ergs/deg. We then divide unit energy, 1.49175×10-3 ergs, by this unit of specific heat, which gives us 7.20423×1012 degrees Kelvin, the natural unit of temperature in the region outside unit distance (that is, for the gaseous state of matter).
We will also be interested in the unit temperature on the T3 basis, the temperature at which the thermal motion reaches the time region boundary. The 3/4 power of 7.20423×1012 is 4.39735×109. But the thermal motion is a motion of matter and involves the 2/9 vibrational addition to the rotationally distributed linear motion of the atoms. This reduces the effective temperature unit by the factor 1 + 2/9, the result being 3.5978×109 degrees K.
On first consideration, this temperature unit may seem incredibly large, as it is far above any observable temperature, and also much in excess of current estimates of the temperatures in the interiors of the stars, which, according to our theoretical findings, can be expected to approach the temperature unit. However, an indication of its validity can be obtained by comparison with the unit of pressure, inasmuch as the temperature and pressure are both relatively simple physical quantities with similar, but opposite, effects on most physical properties, and should therefore have units of comparable magnitude. The conventional units, the degree K and the gram per cubic centimeter have been derived from measurements of the properties of water, and are therefore approximately the same size. Thus the ratio of natural to conventional units should be nearly the same in temperature as in pressure. The value of the temperature unit just calculated, 3.5978×109 degrees K, conforms to this theoretical requirement, as the natural unit of pressure derived in Volume I is 5.386×109 g/cm3.
Except insofar as it enters into the determination of the value of the gas constant, the natural unit of temperature defined for the gaseous state plays no significant role in terrestrial phenomena. Here the unit with which we are primarily concerned is that applicable to the condensed states. Just as the gaseous unit is related to the maximum temperature of the gaseous state, the lower unit is related to the maximum temperature of the the liquid state. This is the temperature level at which the unit molecule escapes from the time region in one dimension of space. The motion in this low energy range takes place in only one scalar dimension. We therefore reduce the three-dimensional unit, 3.5978×109 K, to the one-dimensional basis, and divide it by 3 because of the restriction to one dimension of space. The natural unit applicable to the condensed state is then 1/3 (3.598×109)1/3, degrees K = 510.8 °K.
The magnitude of this unit was evaluated empirically in the course of a study of liquid volume carried out prior to the publication of The Structure of the Physical Universe in 1959. The value derived at that time was 510.2, and this value was used in a series of articles on the liquid state that described the calculation of the numerical values of various liquid properties, including volume, viscosity, surface tension, and the critical constants. Both the 510.2 liquid unit and the gaseous unit were listed in the 1959 publication, but the value of the gaseous unit given there has subsequently increased by a factor of 2 as a result of a review of the original derivation.
Since the basic linear vibrations (photons) of the atom are rotated through all dimensions they have active components in the dimensions of any thermal motion, whatever that dimension may be, just as they have similar components parallel to the rotationally distributed motions. As we found in our examination of the effect on the rotational situation, this basic vibrational component amounts to 2/9 of the primary magnitude. Because the thermal motion is in time (equivalent space) its scalar direction is not fixed relative to that of the vibrational component. This vibrational component will therefore either supplement or oppose the thermal specific heat. The net specific heat, the measured value, is the algebraic sum of the two. This vibrational component does not change the linear relation of the specific heat to the temperature, but it does alter the zero point, as indicated in Figure 2.
Figure 2
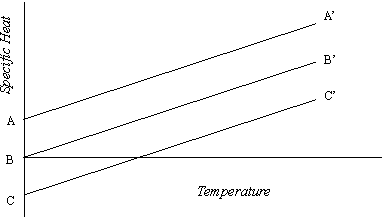
In this diagram the line OB’ is the specific heat curve derived from equation 5-7, assuming a constant value of n and a zero initial level. If the scalar direction of the vibrational component is opposite to that of the thermal motion, the initial level is positive; that is, a certain amount of heat must be supplied to neutralize the vibrational energy before there is any rise in temperature. In this case the specific heat follows the line AA’ parallel to OB’ above it. If the scalar direction of the vibrational component is the same as that of the thermal motion, the initial level is negative, and the specific heat follows the line CC’, likewise parallel to OB’ but below it. Here there is an effective temperature due to the vibrational energy before any thermal motion takes place.
Although this initial component of the molecular motion is effective in determining the temperature, its magnitude cannot be altered and it is therefore not transferable. Consequently, even where the initial level is negative, there is no negative specific heat. Where the sum of the negative initial level and the thermal component is negative, the effective specific heat of the molecule is zero.
It should be noted in passing that the existence of this second, fixed, component of the specific heat confirm the vibrational character of the basic constituent of the atomic structure, the constituent that we have identified as a photon. The demonstration that there is a negative initial level of the specific heat curve is a clear indication of the validity of the theoretical identification of the basic unit in the atomic structure as a vibratory motion.
Equation 5-7 can now be further generalized to include the specific heat contribution of the basic vibration: the initial level, which we will represent by the symbol I. The net specific heat, the value as measured, is then:
|
dH/dT = 2T/n3 +I |
(5-8) |
Where there is a choice between two possible states, as there is between the positive and negative initial levels, the probability relations determine which of the alternatives will prevail. Other things being equal, the condition of least net energy is the most probable, and since the negative initial level requires less net energy for a given temperature than the positive initial level, the thermal motion is based on the negative level at low temperatures unless motion on this basis is inhibited by structural factors.
Addition of energy in the time region takes place by means off a decrease in the effective time magnitude, and it involves eliminating successive time units from the vibration period. The process is therefore discontinuous, but the number of effective time units under ordinary conditions is so large that the relative effect of the elimination of one unit is extremely small. Furthermore, observations of heat phenomena of the solid state do not deal with single molecules but with aggregates of many molecules, and the measurements are averages. For all practical purposes, therefore, we may consider that the specific heat of a solid increases in continuous relation to the temperature, following the pattern defined by equation 5-8.
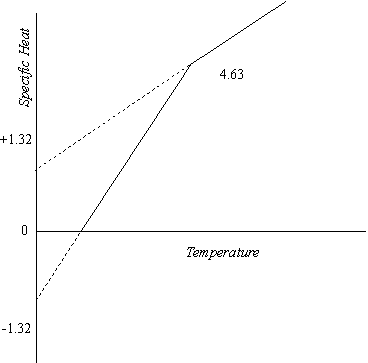
As pointed out earlier in this chapter, the thermal motion cannot cross the time region boundary until its magnitude is sufficient to overcome the progression of the natural reference system without assistance from the gravitational motion; that is, it must attain unit magnitude. The maximum thermal specific heat, the total increment above the initial level, is the value that prevails at the point where the thermal motion reaches this unit level. We can evaluate it by giving each of the terms T and n in equation 5-7 unit value, and on this basis we find that it amounts to 2 natural units, or 3R. The normal initial level is -2/9 and of this 3R is specific heat, or -2/3R. The 3R total is then reached at a net positive specific heat of 2 1/3 R.
Beyond this 3R thermal specific heat level, which corresponds to the regional boundary, the thermal motion leaves the time region and undergoes a change which requires a substantial input of thermal energy to maintain the same temperature, as will be explained later. The condition of minimum energy, the most probable condition, is maintained by avoiding this regional change by whatever means are available. One such expedient, the only one available to molecules in which only one rotational unit is oscillating thermally, is to change from a negative to a positive initial level. Where the initial level is +2/3 R instead of -2/3 R, the net positive specific heat is 3 2/3 R at the point where the thermal specific heat reaches the 3R limit. The regional transmission is not required until this higher level is reached. The resulting specific heat curve is shown in Figure 3.
Inasmuch as the magnetic rotation is the basic rotation of the atom, the maximum number of units that can vibrate thermally is ordinarily determined by the magnetic displacement. Low melting points and certain structural factors impose some further restrictions, and there are a few elements, and a large number of compounds that are confined to the specific heat pattern of Fig.3, or some portion of it. Where the thermal motion extends to the second magnetic rotational unit, to rotation two, we may say, using the same terminology that was employed in the inter-atomic distance discussion, the Fig. 3 pattern is followed up to the 2 1/3 level. At that point the second rotational unit is activated. The initial specific heat level for rotation two is subject to the same n3 factor as the thermal specific heat, and it is therefore 1/n3 x 2/3 R = 1/12 R. This change in the negative initial level raises the net positive specific heat corresponding to the thermal value 3R from 2.333 R to 2.917 R, and enables the thermal motion to continue on the basis of the preferred negative initial level up to a considerably higher temperature.
Figure 3
When the rotation two curve reaches its end point at 2.917 R net positive specific heat, a further reduction of the initial level by a transition to the rotation three basis, where the higher rotation is available, raises the maximum to 2.975 R. Another similar transition follows, if a fourth vibrating unit is possible. The following tabulation shows the specific heat values corresponding to the initial and final levels of each curve. As indicated earlier, the units applicable to the second column under each heading are calories per gram mole per degree Kelvin.
| Vibrating Units |
Effective Initial Level | Maximum Net Specific Heat (negative initial level) |
||
|---|---|---|---|---|
| 1 | -0.667 R | -1.3243 | 2.3333 R | 4.6345 |
| 2 | -0.0833 R | -0.1655 | 2.9167 R | 5.7940 |
| 3 | -0.0247 R | -0.0490 | 2.9753 R | 5.9104 |
| 4 | -0.0104 R | -0.0207 | 2.9896 R | 5.9388 |
Ultimately the maximum net positive specific heat that is possible on the basis of a negative initial level is attained. Here a transition to a positive initial level takes place, and the curve continues on to the overall maximum. As a result of this mechanism of successive transitions, each number of vibrating units has its own characteristic specific heat curve. The curve for rotation one has already been presented in Figure 3. For convenient reference we will call this a type two curve. The different type one curves, those of two, three, and four vibrating units, are shown in Figure 4. As can be seen from these diagrams, there is a gradual flattening and an increase in the ratio of temperature to specific heat as the number of vibratory units increases. The actual temperature scale of the curve applicable to any particular element or compound depends on the thermal characteristics of the substance, but the relative temperature scale is determined by the factors already considered, and the curves in Figure 4 have been drawn on this relative basis.
Figure 4
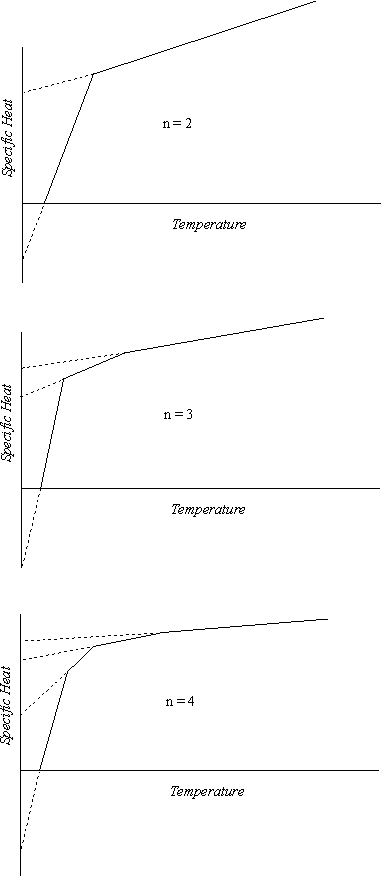
As indicated by equation 5-8, the slope of the rotation two segment of the specific heat curve is only one-eighth of the slope of the rotation one segment. While this second segment starts at a temperature corresponding to 2 1/3 R specific heat, rather than from zero temperature, the fixed relation between the two slopes means that a projection of the two-unit curve back to zero temperature always intersects the zero temperature ordinate at the same point regardless of the actual temperature scale of the curve. The slopes of the three-unit and four-unit curves are likewise specifically related to those of the earlier curves, and each of these higher curves also has a fixed initial point. We will find this feature very convenient in analyzing complex specific heat curves, as each experimental curve can be broken down into a succession of straight lines intersecting the zero ordinate at these fixed points, the numerical values of which are as follows:
| Vibrating Units |
Specific Heat at 0º K (projected) | |
|---|---|---|
| 1 | -0.6667 R | -1.3243 |
| 2 | 1.9583 R | 3.8902 |
| 3 | 2.6327 R | 5.2298 |
| 4 | 2.8308 R | 5.6234 |
These values and the maximum net specific heats previously calculated for the successive curves enable us to determine the relative temperatures of the various transition points. In the rotation three curve, for example, the temperatures of the first and second transition points are proportional to the differences between their respective specific heats and the 3.8902 initial level of the rotation two segment of the curve, as both of these points lie on this line. The relative temperatures of any other pair of points located on the same straight line section of any of the curves can be determined in a similar manner. By this means the following relative temperatures have been calculated, based on the temperature of the first transition point as unity.
| Vibrating Units |
Relative Temperature Transition Point |
End Point |
|---|---|---|
| 1 | 1.000 | 1.80 |
| 2 | 2.558 | 4.56 |
| 3 | 3.086 | 9.32 |
| 4 | 3.391 | 17.87 |
The curves of Figures 3 and 4 portray what may be called the “regular” specific heat patterns of the elements. These are subject to modifications in certain cases. For instance, all of the electronegative elements with displacements below 7 thus far studied substitute an initial level of -0.66 for the normal -1.32. Another common deviation from the regular pattern involves a change in the temperature scale of the curve at one of the transition points, usually the first. For reasons that will be developed later, the change is normally downward. Inasmuch as the initial level of each segment of the curve remains the same, the change in the temperature scale results in an increase in the slope of the higher curve segment. The actual intersection of the two curve segments involved then takes place at a level above the normal transition point.
There are some deviations of a different nature in the upper portions of the curves where the temperatures are approaching the melting points. These will not be given any consideration at this time because they are connected with the transition to the liquid state and can be more conveniently examined in connection with the discussion of liquid properties.
As mentioned earlier, the quantity with which this and the next two chapters are primarily concerned is the specific heat at zero external pressure. In Chapter 6 the calculated values of this quantity will be compared with measured values of the specific heat at constant pressure, as the difference between the specific heat at zero pressure and that at the pressures of observation is negligible. Most conventional theory deals with the specific heat at constant volume rather than at constant pressure, but our analysis indicates that the measurement under constant pressure corresponds to the fundamental quantity.
