CHAPTER 9
Electric Currents
Another set of properties of matter that we will want to consider results from the interaction between matter and one of the sub-atomic particles, the electron. As pointed out in Volume I, the electron, M 0-0-(1), in the notation used in this work, is a unique particle. It is the only particle constructed on the material rotational base, M 0-0-0, (negative vibration and positive rotation) that has an effective negative rotational displacement. More than one unit of negative rotation would exceed the one positive rotational unit of the rotational base, and would result in a negative value of the total rotation. Such a rotation of the basic negative vibration would be unstable in the material environment, for reasons that were explained in the previous discussion. But in the electron the net total rotation is positive, even though it involves one positive and one negative unit, as the positive unit is two-dimensional while the negative unit is one-dimensional.
Furthermore, the independent one-dimensional nature of the rotation of the electron and its positive counterpart, the positron, leads to another unique effect. As we found in our analysis of the rotations that are possible for the basic vibrating unit, the primary rotation of atoms and particles is two-dimensional. The simplest primary rotation has a one-unit magnetic (two-dimensional) displacement, a unit deviation from unit speed, the condition of rest in the physical universe. The electric (one-dimensional) rotation, we found, is not a primary rotation, but merely one that modifies a previously existing two-dimensional rotation. Addition of the one-unit space displacement of the electron rotation to an existing effective two-dimensional rotation increases the total scalar speed of that rotation. But the one-dimensional rotation of the independent electron does not modify an effective speed; it modifies unit speed, which is zero from the effective standpoint. The speed displacement of the independent electron, its only effective component, therefore modifies only the effective space, not the speed.
Thus the electron is essentially nothing more than a rotating unit of space. This is a concept that is rather difficult for most of us when it is first encountered, because it conflicts with the idea of the nature of space that we have gained from a long-continued, but uncritical, examination of our surroundings. However, the history of science is full of instances where it has been found necessary to recognize that a familiar, and apparently unique, phenomenon is merely one member of a general class, all members of which have the same physical significance. Energy is a good example. To the investigators who were laying the foundation of modern science in the Middle Ages the property that moving bodies possess by reason of their motion—“impetus” to those investigators; “kinetic energy” to us—was something of a unique nature. The idea that a motionless stick of wood contained the equivalent of this “impetus” because of its chemical composition was as foreign to them as the concept of a rotating unit of space is to most individuals today. But the discovery that kinetic energy is only one form of energy in general opened the door to a major advance in physical understanding. Similarly, the finding that the “space” of our ordinary experience, extension space, as we are calling it in this work, is merely one manifestation of space in general opens the door to an understanding of many aspects of the physical universe, including the phenomena connected with the movement of electrons in matter.
In the universe of motion, the universe whose details we are developing in this work, and whose identity with the observed physical universe we are demonstrating as we go along, space enters into physical phenomena only as a component of motion, and the specific nature of that space is, for most purposes, irrelevant, just as the particular kind of energy that enters into a physical process usually has no relevance to the outcome of the process. The status of the electron as a rotating unit of space therefore gives it a very special role in the physical activity of the universe. It should be noted at this time that the electron that we are now discussing carries no charge. It is a combination of two motions, a basic vibration and a rotation of the vibrating unit. As we will see later, an electric charge is an additional motion that may be superimposed on this two-component combination. The behavior of charged electrons will be considered after some further groundwork has been laid. For the present we are concerned only with the uncharged electrons.
As a unit of space, the uncharged electron cannot move through extension space, since the relation of space to space does not constitute motion. But under appropriate conditions it can move through ordinary matter,. inasmuch as this matter is a combination of motions with a net positive, or time, displacement, and the relation of space to time does constitute motion. The present-day view of the motion of electrons in solid matter is that they move through the spaces between the atoms. The resistance to the electron flow is then considered to be analogous to friction. Our finding is that the electrons (units of space) exist in the matter, and move through that matter in the same manner as the movement of matter through extension space.
The motion of the electrons is negative with respect to the net motion of material objects. This is illustrated in the following diagram:
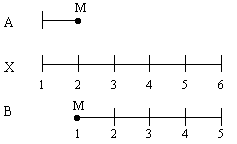
Line X in the diagram is a representation of a scalar magnitude of extension space, as it appears in the conventional reference system. Line A shows the effect of a unit of motion of a material object M through that space. The object that was originally coincident with spatial unit 1 is now coincident with spatial unit 2. Line B shows what happens if the original motion of object M is followed by a unit of electron motion. Just as object M moved through space X in line A, so space X (the electrons) moves through object M in line B. In one unit of motion (line A) object M advances from spatial unit 1 to spatial unit 2. In the following unit of the inverse type of motion (line B) the numbered spatial locations advance one unit relative to object M. This brings M back into coincidence with spatial unit 1, the same result that would have followed if object M had moved backward in the absence of any electron movement. Thus the movement of space (electrons) through matter is equivalent to a negative movement of matter through space. It follows that the voltage differential that causes the electron motion, and the stress in any substance that absorbs the motion, are likewise negative.
Directional movement of electrons through matter will be identified as an electric current. If the atoms of the matter through which the current passes are effectively at rest relative to the structure of the solid aggregate as a whole, uniform motion of the electrons (space) through matter has the same general properties as motion of matter through space. It follows Newton’s first law of motion, and can continue indefinitely without addition of energy. This situation exists in the phenomenon known as superconductivity that has been observed experimentally in many substances at very low temperatures. But where the atoms of a material aggregate are in effective motion thermally, movement of electrons through the matter adds to the spatial component of the thermal motion (that is, increases the speed), and thereby imparts energy (heat) to the moving atoms.
The magnitude of the current is measured by the number of electrons (units of space) per unit of time. Units of space per unit of time is the definition of speed, hence the electric current is a speed. From a mathematical standpoint it is immaterial whether a mass is moving through extension space or space is moving through the mass. Thus in dealing with the electric current we are dealing with the mechanical aspects of electricity, and the current phenomena can be described by the same mathematical equations that are applicable to ordinary motion in space, with appropriate modifications for differences in conditions, where such differences exist. It would even be possible to use the same units, but for historical reasons, and as a matter of convenience, a separate system of units is utilized in present-day practice.
The basic unit of current electricity is the unit of quantity. In the natural system it is the spatial aspect of one electron, which has a speed displacement of one unit. Quantity, q, is therefore equivalent to space, s. Energy has the same status in current flow as in the mechanical relations, and has the space-time dimensions t/s. Energy divided by time is power, 1/s. A further division by current, which has the dimensions of speed, s/t, then produces electromotive force (emf) with the dimensions 1/s × t/s = t/s2. These are, of course, the space-time dimensions of force in general.
The term “electric potential” is commonly used as an alternative to emf, but, for reasons to be discussed later, we will not use “potential” in this sense. Where a more convenient term than emf is appropriate, we will use the term “voltage,” symbol V.
Dividing voltage, t/s2, by current, s/t, we obtain t2/s3. This is resistance, symbol R, the only electrical quantity thus far considered that is not equivalent to a familiar mechanical quantity. Its true nature is revealed by an examination of its space-time structure. The dimensions t2/s3 are equivalent to mass, t3/s3, divided by time, t. Resistance is therefore mass per unit time. The relevance of such a quantity can easily be seen when it is realized that the amount of mass entering into the motion of space (electrons) through matter is not a fixed quantity, as it is in the motion of matter through extension space, but a quantity whose magnitude depends on the amount of movement of the electrons. In motion of matter through extension space the mass is constant while the space depends on the duration of the movement. In the current flow the space (number of electrons) is constant while the mass depends on the duration of the movement. If the flow is only momentary, each electron may move through only a small fraction of the total amount of mass in the circuit, whereas if it continues for a longer time the entire circuit may be traversed repeatedly. In either case, the total mass involved in the current flow is the product of the mass per unit time (the resistance) and the time of flow. In the movement of matter through extension space, the total space is determined in the same manner; that is, it is the product of the space per unit time (velocity) by the time of movement.
In dealing with resistance as a property of matter we will be interested mainly in the specific resistance, or resistivity, which is defined as the resistance of a unit cube of the substance under consideration. Resistance is directly proportional to the distance traveled by the current and inversely proportional to the cross-sectional area of the conductor. It follows that if we multiply the resistance by unit area and divide by unit distance we arrive at a quantity with the dimensions t2/s2 that reflects only the inherent characteristics of the material and the environmental conditions (principally temperature and pressure) and is independent of the geometrical structure of the conductor. The reciprocals of resistance and resistivity are conductance and conductivity, respectively.
With the benefit of the clarification of the space-time dimensions of resistance we can now go back to the empirically determined relations between resistance and other electrical quantities, and verify the consistency of the space-time identifications.
- Voltage: V = IR = s/t × t2/s3 = t/s2
- Power: P = I2R = s2/t2 × t2/s3 = 1/s
- Energy: E = I2Rt = s2/t2 × t2/s3 × t = t/s
This energy equation demonstrates the equivalence of the mathematical expressions of the electrical and mechanical phenomena. Since resistance is mass per unit time, the product of resistance and time, Rt, is equivalent to mass, m. The current, I, is a speed, v. The electrical energy expression RtI2 is thus dimensionally equivalent to the kinetic energy expression ½mv2. In other words, the quantity RtI2 is the kinetic energy of the electron motion.
Instead of using resistance, time, and current, we may put the energy expression into terms of voltage, V (equivalent to IR), and quantity, q, (equivalent to It). The expression for the magnitude of the energy (or work) is then W = Vq. Here we have a definite confirmation of the identification of electric quantity as the equivalent of space. Force, as described in one of the standard physics textbooks, is “an explicitly definable vector quantity that tends to produce a change in the motion of objects.” Electromotive force, or voltage, conforms to this description. It tends to cause motion of the electrons in the direction of the voltage gradient. Energy in general is the product of force and distance. Electrical energy, as Vq, is the product of force and quantity. It follows that electrical quantity is equivalent to distance: the same conclusion that we derived from the nature of the uncharged electron.
In conventional scientific thought the status of electrical energy as one form of energy in general is accepted, as it must be, since it can be converted to any of the other forms, but the status of electrical, or electromotive, force as one form of force in general is not accepted. If it were, the conclusion stated in the preceding paragraph would be inescapable. But the clear verdict of the observed facts is disregarded because there is a general impression that electrical quantity and space are entities of a totally different nature.
The early investigators of electrical phenomena recognized that the quantity measured in volts has the characteristics of a force, and they named it accordingly. Contemporary theorists reject this identification because it conflicts with their views as to the nature of the electric current. W. J. Duffin, for instance, gives us a definition of electromotive force (emf), and then says,
In spite of its name, it is clearly not a force but is equal to the work done per unit positive charge in taking a charge completely around [the electric circuit]; its unit is therefore the volt.13
Work per unit of space is force. This author simply takes it for granted that the moving entity, which he calls a charge, is not equivalent to space, and he therefore deduces that the quantity measured in volts cannot be a force. Our finding is that his assumptions are wrong, that the moving entity is not a charge, but is a rotating unit of space (an uncharged electron). The electromotive force, measured in volts, is then, in fact, a force. In effect, Duffin concedes this point when he tells us, in another connection, that “V/n [volts per meter] is the same as N/C [newtons per coulomb].”14 Both express the voltage gradient in terms of force divided by space.
Conventional physical theory does not pretend to give us any understanding of the nature of either electrical quantity or electric charge. It simply assumes that inasmuch as scientific investigation has hitherto been unable to produce any explanation of its nature, the electric charge must be a unique entity, independent of the other fundamental physical entities, and must be accepted as one of the “given” features of nature. It is then further assumed that this entity of unknown nature that plays the central role in electrostatic phenomena is identical with the entity of unknown nature, electrical quantity, that plays the central role in current electricity.
The most significant weakness of the conventional theory of the electric current, the theory based on the foregoing assumptions, as we now see it in the light of the more complete understanding of physical fundamentals derived from the theory of the universe of motion, is that it assigns two different, and incompatible, roles to the electrons. These particles, according to present-day theory, are components of the atomic structure, yet at least some of them are presumed to be free to accommodate themselves to any electrical forces applied to the conductor. On the one hand, each is so firmly bound to the remainder of the atom that it plays a significant part in determining the properties of that atom, and a substantial force (the ionization potential) must be applied in order to separate it from the atom. On the other hand, these electrons are so free to move that they will respond to thermal or electrical forces whose magnitude is only slightly above zero. They must exist in a conductor in specific numbers in order to account for the fact that the conductor is electrically neutral while carrying current, but at the same time they must be free to leave the conductor, either in large or small quantities, if they acquire sufficient kinetic energy.
It should be evident that the theories are calling upon the electrons to perform two different and contradictory functions. They have been assigned the key position in both the theory of atomic structure and the theory of the electric current, without regard for the fact that the properties that they must have in order to perform the functions required by either one of these theories disqualify them for the functions that they are called upon to perform in the other.
In the theory of the universe of motion, each of these phenomena involves a different physical entity The unit of atomic structure is a unit of rotational motion, not an electron. It has the quasi-permanent status that is required of an atomic constituent. The electron, without a charge, and without any connection with the atomic structure, is then available as the freely moving unit of the electric current.
The fundamental postulate of the Reciprocal System of theory is that the physical universe is a universe of motion, one in which all entities and phenomena are motions, combinations of motions, or relations between motions. In such a universe none of the basic phenomena are unexplainable. “Unanalyzables,” as Bridgman called them, do not exist. The basic physical entities and phenomena of the universe of motion—radiation, gravitation, matter, electricity, magnetism, and so on—can be defined explicitly in terms of space and time. Unlike conventional physical theory, the Reciprocal System does not have to leave its basic elements cloaked in metaphysical mystery. It does not have to exclude them from physical inquiry, in the manner of the following statement from the Encyclopedia Britannica:
The question “What is electricity?” like the question “What is matter?” really lies outside the realm of physics and belongs to that of metaphysics.15
In a universe composed entirely of motion, an electric charge applied to a physical entity must necessarily be a motion. Thus the problem faced in the theoretical investigation was not to answer the question, What is an electric charge?, but merely to determine what kind of motion manifests itself as a charge. The identification of the charge as an added motion not only clarifies the relation between the charged electron that is observed experimentally and the uncharged electron that is known only as the moving entity in the electric current, but also explains the interchanges between the two that are the principal support for the currently popular opinion that only one entity, the charge, is involved. It is not always remembered that this opinion achieved general acceptance only after a long and spirited controversy. There are similarities between static and current phenomena, but there are also significant differences. Inasmuch as no theoretical explanation of either kind of electric effect was available at the time, the question to be decided was whether to regard the two as identical because of the similarities, or as disparate because of the differences. Once made, the decision in favor of identity has persisted, even though much evidence against its validity has accumulated in the meantime.
The similarities are of two general types: (1) some of the properties of charged particles and electric currents are alike, and (2) there are observable transitions from one to the other. Identification of the charged electron as an uncharged electron with an added motion explains both types of similarities. For instance, a demonstration that a rapidly moving charge has the same magnetic properties as an electric current was a major factor in the victory won by the proponents of the “charge” theory of the electric current many years ago. But our findings are that the moving entities are electrons, or other carriers of the charges, and the existence or non-existence of electric charges is irrelevant.
The second kind of evidence that has been interpreted as supporting the identity of the static and current electrons is the apparent continuity from the electron of current flow to the charged electron in such processes as electrolysis. Here the explanation is that the electric charge is easily created and easily destroyed. As everyone knows, nothing more than a slight amount of friction is sufficient to generate an electric charge on many surfaces, such as those of present-day synthetic fabrics. It follows that wherever a concentration of energy exists in one of these forms that can be relieved by conversion to the other, the rotational vibration that constitutes a charge is either initiated or terminated in order to permit the type of electron motion that can take place in response to the effective force.
It has been possible to follow the prevailing policy, regarding the two different quantities as identical, and utilizing the same units for both, only because the two different usages are entirely separate in most cases. Under these circumstances no error is introduced into the calculations by using the same units, but a clear distinction is necessary in any case where either calculation or theoretical consideration involves quantities of both kinds.
As an analogy we might assume that we are undertaking to set up a system of units in which to express the properties of water. Let us further assume that we fail to recognize that there is a difference between the properties of weight and volume, and consequently express both in cubic centimeters. Such a system is equivalent to using a weight unit of one gram, and as long as we deal separately with weight and volume, each in its own context, the fact that the expression “cubic centimeter” has two entirely different meanings will not result in any difficulties. However, if we have occasion to deal with both quantities simultaneously, it is essential to recognize the difference between the two. Dividing cubic centimeters (weight) by cubic centimeters (volume) does not result in a pure number, as the calculations seem to indicate; the quotient is a physical quantity with the dimensions weight/volume. Similarly, we may use the same units for electric charge and electric quantity as long as they are employed independently and in the right context, but whenever the two enter in to the same calculation, or are employed individually with the wrong physical dimensions, there is confusion.
This dimensional confusion resulting from the lack of distinction between the charged and uncharged electrons has been a source of considerable concern, and some embarrassment to the theoretical physicists. One of its major effects has been to prevent setting up any comprehensive systematic relationship between the dimensions of physical quantities. The failure to find a basis for such a relationship is a clear indication that something is wrong in the dimensional assignments, but instead of recognizing this fact, the current reaction is to sweep the problem under the rug by pretending that it does not exist. As one observer sees the picture:
In the past the subject of dimensions has been quite controversial. For years unsuccessful attempts were made to find “ultimate rational quantities” in terms of which to express all dimensional formulas. It is now universally agreed that there is no one “absolute” set of dimensional formulas.16
This is a very common reaction to long years of frustration, one that we encountered frequently in our examination of the subjects treated in Volume I. When the most strenuous efforts by generation after generation of investigators fail to reach a defined objective, there is always a strong temptation to take the stand that the objective is inherently unattainable. “In short,” says Alfred Lande, “if you cannot clarify a problematic situation, declare it to be ‘fundamental,’ then proclaim a corresponding ‘principle’.”17 So physical science fills up with principles of impotence rather than explanations.
In the universe of motion the dimensions of all quantities of all kinds can be expressed in terms of space and time only. The space-time dimensions of the basic mechanical quantities were identified in Volume I. In this chapter we have added those of the quantities involved in the flow of electric current. The chapters that follow will complete this task by identifying the space-time dimensions of the electric and magnetic quantities that make their appearance in the phenomena due to charges of one kind or another and in the magnetic effects of electric currents.
This clarification of the dimensional relations is accompanied by a determination of the natural unit magnitudes of the various physical quantities. The system of units commonly utilized in dealing with electric currents was developed independently of the mechanical units on an arbitrary basis. In order to ascertain the relation between this arbitrary system and the natural system of units it is necessary to measure some one physical quantity whose magnitude can be identified in the natural system, as was done in the previous determination of the relations between the natural and conventional units of space, time, and mass. For this purpose we will use the Faraday constant, the observed relation between the quantity of electricity and the mass involved in electrolytic action. Multiplying this constant, 2.89366×1014 esu/g-equiv., by the natural unit of atomic weight, 1.65979×10-24 g, we arrive at 4.80287×10-10 esu as the natural unit of electrical quantity.
The magnitude of the electric current is the number of electrons per unit of time; that is, units of space per unit of time, or speed. Thus the natural unit of current could be expressed as the natural unit of speed, 2.99793×1010 cm/sec. In electrical terms it is the natural unit of quantity divided by the natural unit of time, and amounts to 3.15842×106 esu/sec, or 1.05353×10-3 amperes. The conventional unit of electrical energy, the watt-hour, is equal to 3.6×1010 ergs. The natural unit of energy, 1.49275×10-3 ergs, is therefore equivalent to 4.14375×10-14 watt-hours. Dividing this unit by the natural unit of time, we obtain the natural unit of power
9.8099×1012 ergs/sec = 9.8099×105 watts. A division by the natural unit of current then gives us the natural unit of electromotive force, or voltage, 9.31146×108 volts. Another division by current brings us to the natural unit of resistance, 8.83834×1011 ohms.
The basic quantities of current electricity and their natural units in electrical terms can be summarized as follows:
| s | quantity |
4.80287×10-10 esu |
|---|---|---|
| s/t | current | 1.05353×10-3 amperes |
| 1/s | power | 9.8099×105 watts |
| t/s | energy | 4.14375×10-14 watt-hours |
| t/s2 | voltage | 9.31146×108volts |
| t2/s3 | resistance | 8.83834×1011 ohms |
Another electrical quantity that should be mentioned because of the key role that it plays in the present-day mathematical treatment of magnetism is “current density,” which is defined as “the quantity of charge passing per second through unit area of a plane normal to the line of flow.” This is a strange quantity, quite unlike any physical quantity that has previously been discussed in the pages of this and the preceding volume, in that it is not a relation between space and time. When we recognize that the quantity is actually current per unit of area, rather than “charge” (a fact that is verified by the units, amperes per square meter, in which it is expressed), its space-time dimensions are seen to be s/t × 1/s2 = 1/st. These are not the dimensions of a motion, or a property of a motion. It follows that this quantity, as a whole, has no physical significance. It is merely a mathematical convenience.
The fundamental laws of current electricity known to present-day science—such as Ohm’s Law, Kirchhoff’s Laws, and their derivatives—are empirical generalizations, and their application is not affected by the clarification of the essential nature of the electric current. The substance of these laws, and the relevant details, are adequately covered in existing scientific and technical literature. In conformity with the general plan of this work, as set forth earlier, these subjects will not be included in our presentation.
This is an appropriate time to make some comments about the concept of “natural units.” There is no ambiguity in this concept, so far as the basic units of motion are concerned. The same is true, in general, of the units of the simple scalar quantities, although some questions do arise. For example, the unit of space in the region inside unit distance, the time region, as we are calling it, is inherently just as large as the unit of space in the region outside unit distance, but as measured it is reduced by the inter-regional ratio, 156.444, for reasons previously explained. We cannot legitimately regard this quantity as something less than a full unit, since, as we saw in Volume I, it has the same status in the time region that the full-sized natural unit of space has in the region outside unit distance. The logical way of handling this situation appears to be to take the stand that there are two different natural units of distance (one-dimensional space), a simple unit and a compound unit, that apply under different circumstances.
The more complex physical quantities are subject to still more variability in the unit magnitudes, because these quantities are combinations of the simpler quantities, and the combination may take place in different ways and under different conditions. For instance, as we saw in our examination of the units of mass in Volume I, there are several different manifestations of mass, each of which involves a different combination of natural units and therefore has a natural unit of its own. In this case, the primary cause of variability is the existence of a secondary mass component that is related to the primary mass by the inter-regional ratio, or a modification thereof, but some additional factors introduce further variability, as indicated in the earlier discussion.
