CHAPTER 6
The Dwarf Star Cycle
At the very high temperatures prevailing in the interiors of the stars at the upper end of the main sequence the thermal velocities are approaching the unit level, and when these already high velocities are further increased by the energy released in the supernova explosion the speeds of many of the interior atoms rise above unity. The results of speeds above the unit level were discussed briefly in Volume I, but a more detailed consideration will now be required, as these greater-than-unit speeds, which play no part in the physical activity of our terrestrial environment, are involved in a wide variety of astronomical phenomena.
The discovery of the existence of speeds greater than that of light is one of the most significant results of the development of the theory of the universe of motion. It has opened the door to an understanding of many previously obscure or puzzling phenomena and relations. But some of the concepts that are involved in dealing with these very high speeds are new and unfamiliar. For that reason many persons find them hard to accept on the strength of theoretical reasoning alone, regardless of how solid a base that reasoning may have. The results of recent research reported in The Neglected Facts of Science, published in 1982, should be very helpful to these individuals, as that research has shown that many of the new findings derived from the theory of the universe of motion can also be derived from purely factual premises, independently of any theory, thus providing an empirical validation of the theoretical results. Among these theoretical conclusions that are now provided with factual proof are the items with which we are presently concerned: the existence of greater-than-unit speeds, and the characteristics of motion at these speeds. In order to emphasize the point that the theoretical findings in this areas however strange they may appear in the light of previously accepted ideas, are fully confirmed by observed facts and logical deductions from those facts, the description of the basic motions of the universe, for purposes of the theoretical development in this work, has been taken from the purely factual derivation given in the 1982 publication.
This factual development was made possible by recognition of the physical evidence of the existence of scalar motion, and a detailed analysis of the properties of motion of this nature The scalar nature of the basic motions of the universe is an essential feature of the Reciprocal System of theory, and has been emphasized from the time of its first presentation. The points brought out in the extract from the 1982 book are simply the necessary consequences of the existence of these basic scalar motions. However, in order to follow the development of thought, it will be necessary to bear in mind some of the special features of scalar motion that were brought out in the previous volumes of this work. Although scalar motion, by definition, has no direction, in the usual sense of that term, it can be either positive or negative. When such motions are represented in a reference system, the positive and negative magnitudes appear as outward and inward respectively. For convenient reference, these are designated as “scalar directions.” Inasmuch as a scalar motion is simply the relation between a space magnitude and a time magnitude, it can be measured either as speed, the relation of space to time, or as inverse speed, the relation of time to space. Inverse speed was identified, in Volume I, as energy. A reciprocal relation, such as that between space and time in motion, is symmetrical about the unit value; that is, speeds of l/n (which we have identified as motion in space) are equivalent to inverse speeds, or energies, of n/l, whereas energies of l/n (which we have identified as motion in time) are equivalent to speeds of n/l. With the benefit of this understanding of those of the relevant factors that may be unfamiliar, we may now begin the extract from the published description of the high-speed regions.
Photons of radiation have no capability of independent motion, and are carried outward at unit speed by the progression of the natural reference system, as shown in (I), Figure 7. All physical objects are moving outward in the same manner, but those objects that are subject to gravitation are coincidentally moving inward in opposition to the outward progression. When the gravitational speed of such an object is unity, and equal to the speed of progression of the natural reference system, the net speed relative to the fixed spatial reference system is zero, as indicated in (2). In (3) we see the situation at the maximum gravitational speed of two units. Here the net speed reached is -1, which, by reason of the discrete unit limitation, is the maximum, in the negative direction.

An object moving with speed combination (2) or (3) can acquire a translational motion in the outward scalar direction. One unit of the outward translational motion added to combination (3) brings the net speed relative to the fixed reference system, combination (4), to zero. Addition of one more translational unit, as in combination (5), reaches the maximum speed, +1, in the positive scalar direction. The maximum range of the equivalent translational speed in any one scalar dimension is thus two units.
As indicated in Figure 7, the independent translational motions with which we are now concerned are additions to the two basic scalar motions, the inward motion of gravitation and the outward progression of the natural reference system. The net speed after a given translational addition therefore depends on the relative strength of the two original components, as well as on the size of the addition. That relative strength is a function of the distance. The dependence of the gravitational effect on distance is well known. What has not heretofore been recognized is that there is an opposing motion (the outward progression of the natural reference system) that predominates at great distances, resulting in a net outward motion.
The outward motion (recession) of the distant galaxies is currently attributed to a different cause, the hypothetical Big Bang, but this kind of an ad hoc assumption is no longer necessary. Clarification of the properties of scalar motion has made it evident that this outward motion is something in which all physical objects participate. The outward travel of the photons of radiation, for instance, is due to exactly the same cause. Objects such as the galaxies that are subject to gravitation attain a full unit of net speed only where gravitation has been attenuated to negligible levels by extreme distances. The net speed at the shorter distances is the resultant of the speeds of the two opposing motions. As the distance decreases from the extreme values, the net outward motion likewise decreases, and at some point, the gravitational limit, the two motions reach equality, and the net speed is zero. Inside this limit there is a net inward motion, with a speed that increases as the effective distance decreases. Independent translational motions, if present, modify the resultant of the two basic motions.
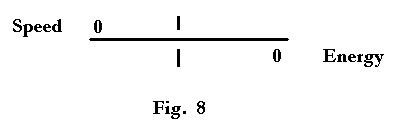
The units of translational motion that are applied to produce the speeds in the higher ranges are outward scalar units superimposed on the motion equilibria that exist at speeds below unity, as shown in combination (5), Figure 7. The two-unit maximum range in one dimension involves one unit of speed, s/t, extending from zero speed to unit speed, and one unit of inverse speed, t/s, extending from unit speed to zero inverse speed. Unit speed and unit energy (inverse speed) are equivalent, as the space-time ratio is 1/1 in both cases, and the natural direction is the same; that is, both are directed toward unity, the datum level of scalar motion. But they are oppositely directed when either zero speed or zero energy is taken as the reference level. Zero speed and zero energy in one dimension are separated by the equivalent of two full units of speed (or energy) as indicated in Figure 8.
In the foregoing paragraphs we have been dealing with full units. In actual practice, however, most speeds are somewhere between the unit values. Since fractional units do not exist, these speeds are possible only because of the reciprocal relation between speed and energy, which makes an energy of n/l equivalent to a speed of l/n. While a simple speed of less than one unit is impossible, a speed in the range below unity can be produced by addition of units of energy to a unit of speed. The quantity 1 /n is modified by the conditions under which it exists in the spatial reference system (for reasons explained in the earlier volumes), and appears in a different mathematical form, usually l/n2.
Since unit speed and unit energy are oppositely directed when either zero speed or zero energy is taken as the reference level, the scalar direction of the equivalent speed 1/n2 produced by the addition of energy is opposite to that of the actual speed, and the net speed in the region below the unit level, after such an addition is 1 - 1/n2. Motion at this speed often appears in combination with a motion 1 - 1/m2 that has the opposite vectorial direction. The net result is then l/n2 - 1/m2, an expression that will he recognized as the Rydberg relation that defines the spectral frequencies of atomic hydrogen—the possible speeds of the hydrogen atom.
The net effective speed 1 - 1/n2 increases as the applied energy n is increased, but inasmuch as the limiting value of this quantity is unity, it is not possible to exceed unit speed (the speed of light) by this inverse process of adding energy. To this extent we can agree with Einstein’s conclusion. However, his assertion that higher speeds are impossible is incorrect, as there is nothing to prevent the direct addition of one or two full units of speed in the other scalar dimensions. This means that there are three speed ranges. Because of the existence of these three ranges with different space and time relationships, it will be convenient to have a specific terminology to distinguish between them. In the subsequent discussion we will use the terms low speed and high speed in their usual significance, applying them only in the region of three-dimensional space, the region in which the speeds are 1 - 1/n2. The region in which the speeds are 2 - 1/n2 that is, above unity, but below two units—will be called the intermediate region, and the corresponding speeds will be designated as intermediate speeds. Speeds in the 3 - 1/n2 range will be called ultra high speeds.
The foregoing paragraphs conclude the portions of the text of The Neglected Facts of Science that are relevant to the intermediate speed range. Consideration of speeds in the ultra high range will be deferred to later sections of this volume, as the phenomena now under review are limited to speeds below two units. However, one point that was mentioned in the extract from the 1982 publication, which should have some further emphasis in view of its importance in the present connection, is the status of unit speed. The true datum level of scalar motion, the physical zero, as we called it in the earlier volumes, is unit speed, not either of the mathematical zero points. This is significant, because it means that the second unit of motion, as measured from zero speed, does not add to the first unit. It replaces that unit. Although the use of zero speed as a reference level makes it appear that the sequence of units is 0, 1, 2, the status of unit speed as the true physical zero means that the correct sequence is -1, 0, +1. The importance of this point is its effect on the second unit of motion. This second unit is not the spatial motion (speed) of the first unit plus a unit of motion in time (energy), but the unit of motion in time only.
The speeds of the fast-moving products of the supernova explosions that we are now undertaking to examine are in the intermediate range, where motion is in time. Instead of being blown outward in space in the same manner as the products that are ejected at speeds below unity, these intermediate speed products are blown outward in time. In both cases, the atoms, which were in relatively close contact in the hot massive star, are widely separated in the explosion product, but in the intermediate speed product the separation is in time rather than in space. This does not change either the mass or the volumetric characteristics of the atoms of matter. But when we measure the density, m/V, of the giant stars we include in V, because of our method of measurement, not only the actual equilibrium volume of the atoms, but also the empty three-dimensional space between the atoms, and the density of the star- calculated on this basis is something of a totally different order from the actual density of the matter of which it is composed.
Similarly, where the atoms are separated by empty time rather than by empty space the volume obtained by our methods of measurement includes the effect of the empty three-dimensional time between the atoms, which reduces the equivalent space (the apparent volume), and again the density calculated in the usual manner has no resemblance to the actual density of the stellar material. In the giant stars the empty space between the atoms (or molecules) decreases the measured density by a factor which may be as great as 105 or l06.The time separation produces a similar effect in the opposite direction, and the second product of the explosion is therefore an object of small apparent volume, but extremely high density: a white dwarf star.
The name “white dwarf” was applied to these stars in the early days just after their discovery, when only a few of them were known. These had temperatures in the white region of the spectrum, and the designation that was given them was intended to distinguish them from the red dwarfs in the lower portions of the main sequence. In the meantime it has been found that the temperature range of these stars extends to much lower levels, leading to the use of such expressions as “red white dwarf.” But by this time the name “white dwarf” is firmly established by usage, and it will no doubt be permanent, even though it is no longer appropriate.
When judged by terrestrial standards, the calculated densities of these white dwarfs are nothing less than fantastic, and the calculations were originally accepted with great reluctance after all alternatives that could be found were ruled out for one reason or another. The indicated density of Sirius B. for instance, is about 130,000 g/cm3, that of Procyon B is estimated at 900,000 g/cm3, while other stars of this type have still greater densities. In the light of the relationships developed in this work, however, it is clear that this very high density is no more out of line than the very low density of the giant stars. Each of these phenomena is simply the inverse of the other. Donald Lynden-Bell expresses the conventional wisdom on the subject in this statement:
We know already that some stars have collapsed to a size only ten times larger than that at which they would become black holes.60
In the face of this asserted “knowledge” it may not be easy to accept the idea that these objects have, in fact, expanded to their present size; that is, their components have moved outward away from each other in time, and the small size that we observe is merely a result of the way in which the expansion in time appears in the spatial reference system. But this conclusion is a necessary consequence of basic physical principles whose validity has been demonstrated in the preceding volumes of this series, and, as we will see in the subsequent pages, it produces explanations of the white dwarf properties that are in full agreement with all of the definitely established observational information.
Unfortunately, the amount of observational information with respect to the white dwarfs that has been accumulated thus far is very limited, and much of what is available is of questionable accuracy. This scarcity of reliable information is due to a combination of causes. The white dwarfs have been known for only a relatively short time. The first one to be seen, the “pup” companion of Sirius, the dog star, was observed in 1862, but it was not until about 1915 that the distinctive character of the properties of this star was recognized, and theories to account for these properties were not developed until considerably later. The second reason for the lack of information is the dimness of these objects, which makes them very difficult to see, and limits both the number of stars that can be observed and the amount of information that can be obtained from each.
The third factor that has led to confusion in this area is the lack of a correct theoretical explanation of the white dwarf structure. As indicated in the statement quoted above, the currently accepted theory envisions an atomic collapse. It is asserted that the energy supply of a star is eventually exhausted, and that when energy generation ceases, the star collapses into a hypothetical state called “degenerate matter” in which the space between the hypothetical constituents of the atoms is eliminated, and these constituents are squeezed into a close-packed condition. As explained by Robert Jastrow:
With its fuel gone it [the star] can no longer generate the pressures needed to maintain itself against the crushing force of gravity, and it begins to collapse once more under its own weight.61
Joseph Silk’s explanation is essentially the same:
The pressure exerts an outward force that withstands the gravity of the star, as long as there is sufficient hydrogen present in the stellar core to produce helium…
After the supply of nuclear energy runs out and fails to provide adequate heat and pressure, gravitational collapse must ensue.62
This is an astounding conclusion. To put it into the proper perspective, it should be realized that the hypothetical collapse is something that is supposed to take place within the atom; that is, the pressure exerted on the atoms becomes so great that they are unable to withstand it. But, in fact, the pressure to which the atoms of the condensed gas are subjected to is not significantly altered by the cooling that results when and if the energy generation ceases. Each atom is subject to the pressure due to the weight of all overlying matter in any event, regardless of whether that matter is hot or cold. The pressure due to the thermal motion has nothing to do with conditions inside the atom; it merely introduces additional space between the atoms. Certainly, this added space would be eliminated if the star cooled down by reason of the exhaustion of the energy supply, but this would not change the conditions to which the atoms are subjected.
The books from which an earlier generation of Americans learned to read contained a story about a man who was returning home from the city with a heavy sack of flour that he had purchased. He was afraid that the weight of the flour would be too much for the horse that he was riding, so to lighten the load on the horse he held the sack in his arms. In those days, the children that read the story found it hilarious, but now we are confronted with essentially the same thing in different language, and we are expected to take it seriously.
Some writers seem to suggest the existence of a kinetic component that would add to the static pressure against the central atoms. Paolo Maffei gives us this version of the “collapse”:
Eventually, when all the lighter energy-producing elements have been depleted, energy will no longer be generated in the interior of the sun. In the absence of the internal pressure that supported them, the outer shells will rapidly fall toward the center due to gravitational attraction. In the course of this very rapid collapse, the atoms will be squeezed together ever more tightly, and the electrons will be disassociated from the nuclei.63
But the assumption that a star could cool down rapidly enough to increase the total pressure significantly is nothing short of outrageous. There is no reason to believe that the heat transfer process within the star will be any faster during the cooling process than in the normal outward flow. Indeed, the cooling will be slowed up considerably by the release of gravitational energy as the outer portions of the star move inward. Furthermore, even under the most extreme assumptions, the critical pressure at which the atomic collapse is presumed to occur could be reached only in the very large stars, since the central atoms in the smaller stars can obviously withstand pressures immensely greater than the static pressure to which they are normally subject. (We know this to be true because atoms of the same kind do withstand these immensely greater pressures in the large stars). Thus the collapse, if it occurred at all, could occur only in the stars which current theory says do not collapse, but explode. And no one bothers to explain how the layers of matter outside the central regions of the star, which certainly are not subjected to any excessive pressure, are induced to participate in the degeneracy.
The truth is that the question as to how matter gets from its normal state into this hypothetical degenerate condition is given scant attention. The astronomers have arrived at an explanation of the extremely high density of the white dwarfs that appears reasonable in the context of the currently accepted theory of atomic structure. That theory portrays the atoms in terms of individual constituents separated by large amounts of space. Elimination of this space seems to be a logical way of accounting for the enormous increase in density. No direct evidence bearing on this issue is currently available, and the hypothesis is therefore free from any direct conflict with observation. Having this (to them) satisfactory explanation of the density of the white dwarf, the astronomers have apparently considered it obvious that the stars must get from their normal condition to this white dwarf state in some way. Consequently, they have not considered it necessary to look very closely into the question as to how the collapse is to be accomplished.
Eddington is often credited with having provided the “explanation” of the white dwarfs.64 But an examination of one of his discussions of the subject, such as that in the chapter on “The Constitution of the Stars” in his New Pathways in Science,65 reveals that the whole point of his discussion is to show that the existence of degenerate matter is consistent with accepted atomic theory. He does not address the question as to how this degeneracy is to be accomplished, except to comment that it can be produced by pressure, which gets us nowhere, as he offers no suggestion as to how the necessary pressure could be produced—the same lacuna that is so evident in the more recent discussions of the subject. Where such a suggestion is attempted, it is usually an obvious absurdity. Here is an example:
Gravitation tends to squeeze the star to smaller and smaller dimensions, but every contraction only strengthens the force, thereby compelling further contraction… Its [the star’s] contraction accelerates all the time for the reasons just explained, and outright would collapse into a black hole if forces were not generated to counteract the gravitational contraction. Such a force is the thermal pressure of the gas… the pressure eventually begins to balance gravitation.66 (M. J. Plavec)
This not only conflicts with the previously noted fact that the thermal pressure does not alter the pressure exerted against the atoms, but is also specifically contradicted by direct observation, as we know from experience that matter in which thermal pressure is not “generated to counteract the gravitational contraction,”—that is, matter that is near zero absolute temperature—does not “collapse into a black hole.” It remains in the condition that we call the solid state, in which there is a definite minimum distance between the atoms. This is an equilibrium distance, and it can be reduced by application of pressure, but there is no observational indication of any kind of a limit, even though pressures as high as five million atmospheres have been reached in experiments.
The truth is that there is no empirical evidence to support the assumption that gravitation operates within atoms. Observations show only that there is a gravitational effect between atoms (and other discrete particles). Furthermore, the behavior of matter under compression demonstrates that there is a counterforce, an antagonist to gravitation (the same) that we encountered earlier in our examination of the structure of the globular clusters) that limits the extent to which the gravitational force can decrease the inter-atomic distance. Plavec’s contention that collapse into a black hole will take place unless forces, such as the thermal pressure, are “generated” to oppose gravitation is contradicted by the observed behavior of matter, which shows that the necessary counter-force is inherent in the structure of matter itself, and does not have to be generated by a supplementary process.
In order to clear the way for the “collapse” hypothesis, it is first necessary to assume that there is a limit to the strength of the counter-force, an assumption that is entirely ad hoc, since current science has not even identified the nature of this force, to say nothing of establishing its limits, if any. Then it is further necessary to assume that the gravitational force operates within the atom and that the opposing force is not so operative to any significant extent. The combination of these latter assumptions is inherently improbable, and in view of the lack of any indication of a limit to the resistance to compression, the first assumption has no more claim to plausibility. The theory of atomic collapse is thus simply an excursion into the realm of the imagination.
In the universe of motion stars cannot and do not collapse. The results that are currently attributed to this hypothetical collapse are produced by the expansion of the fastest products of the supernova explosion into time. The factor that controls the course of development of the white dwarf stars is the inversion of physical properties in the intermediate speed region. As we have seen, the expansion into time increases the amount of three-dimensional time occupied by this star. This is equivalent to a decrease in the volume of space; that is, the equivalent spatial dimensions are reduced, resulting in an increase in density when measured as mass per unit of volume.
Contraction of the matter of the white dwarf star under pressure has the opposite effect, just as it does in the case of ordinary matter. Pressure thus reduces the density measured on this same basis. The constituents of a white dwarf star, like those of any other star, are subject to the gravitational effect of the structure as a whole, and the atoms in the interior are therefore under a pressure. The natural direction of gravitation is always toward unity. In the intermediate region (speeds above unity), as in the time region (distances below unity) that we explored in the earlier volumes, toward unity is outward in the context of a fixed spatial reference system, the datum level of which is zero. Thus the gravitational force in the white dwarf star is inverse relative to the fixed system of reference. It operates to move the atoms closer together in time, which is equivalent to farther apart in space. At the location where the pressure due to the gravitational force is the strongest, the center of the star, the compression in time is the greatest, and since compression in time is equivalent to expansion in space, the center of a white dwarf is the region of lowest density. As we will see later, this inverse density gradient plays an important part in determining the properties of the white dwarfs.
Another effect of the inversion at the unit level can be seen in the relation of the size of the white dwarf to its mass. References are made in the astronomical literature to the “curious” fact that “the more massive a white dwarf is, the smaller its radius.”67 When the true nature of the white dwarf is understood, this is no longer curious. A massive cloud of matter expanding into space occupies more space than one of less mass, and the radius of the massive cloud is therefore greater. A massive cloud of matter expanding into time similarly occupies more time than one of less mass, and the radius of the massive cloud (measured as a spatial quantity) is therefore smaller, inasmuch as more time is equivalent to less space.
Astronomical observations give us only occasional disconnected glimpses of the white dwarf stars as they move through the various stages of their existence, but we can arrive at a theoretical picture of their evolution that is in full agreement with the little that is observationally known. The following paragraphs will outline the general nature of the evolutionary development, which will be considered in detail in Chapters 11, 12, and 13.
In what may be called Stage 1, the immediate post-ejection period following the supernova explosion in which the white dwarf is formed, this star is expanding in time. This means that from a spatial standpoint it is contracting in equivalent space. In this stage, the constituent particles, newly raised to intermediate speeds, are emitting radiation at radio frequencies as they move toward isotopic stability at these speeds. (The process by which the radiation is generated will be examined in Chapter 18). Such a star is observable only as an otherwise unidentifiable source of radio emission. A great many such sources—“blank fields,” as they are known to the observers—have been located, and presumably many of these are outgoing white dwarfs.
During this expansion stage energy is being lost to the environment, and there is little generation of energy to replace the losses. Energy production by atomic disintegration is reduced as the temperature rises in the range above unity, as this decreases the inverse temperature, which determines the destructive limits of the elements in the intermediate speed range. Since unity is the natural datum for physical activity, the critical level at which the disintegration of the atom takes place is unit equivalent temperature, corresponding to the speed of light, regardless of whether the pre-disintegration temperature is above or below the unit level. A deviation upward from unity (a decrease in inverse speed) has the same effect on the process as a downward deviation of the same magnitude (a decrease in speed). Inasmuch as the maximum speed is well above unity, only the very heavy elements are initially available as fuel.
When the energy loss to the environment has been sufficient to terminate the contraction in equivalent space, a process of re-expansion begins. The energy loss continues throughout this second evolutionary stage. As the expansion proceeds, and as the temperature falls toward unity, energy production increases to some extent, since successively lighter elements reach their destructive limits in the same manner as in the inverse situation on the opposite side of the unit temperature level. But the supply of elements heavier than iron was reduced to near zero before the supernova explosion, and the expanding white dwarf therefore has very little fuel for energy generation. The atom building process and the accretion of matter from the environment eventually begin replenishing the supply, but this proceeds at a relatively slow pace. Furthermore, the white dwarf does not have the benefit of gravitational energy, such as that which is released by the contraction of the giant stars, because the effect of gravitation in time is the inverse of the effect of gravitation in space.
Because of the energy losses, the temperatures of the constituents of a white dwarf continually decrease, and eventually they begin dropping below the unit level. As this reversion to the lower speed range proceeds, the star is gradually converted from the status of a white dwarf (a star whose constituents move at intermediate speeds) to that of an ordinary main sequence star (one whose constituents move at speeds below the unit level). The evolution of the white dwarf is thus directed toward the same end as the evolution of the giant stars; that is, a restoration of the state of gravitational and thermal equilibrium that was destroyed by the supernova explosion. In the case of the red giant, the explosion produced a cool and diffuse aggregate, which had to contract and heat in order to reach the equilibrium condition. In the case of the white dwarf, the explosion produced a dense hot aggregate that had to expand and cool in order to reach the same equilibrium condition.
Since the astronomers do not recognize the true nature of the white dwarf star, they have had great difficulty in charting an evolutionary course for these objects. As noted earlier, they have developed a theory of stellar evolution that takes the stars as far as the red giant stage. They regard the white dwarfs as being in the last stage on the road to stellar oblivion. It follows, so they conclude, that the stars must, in some way, get from red giant to white dwarf. The amount of progress that has been made toward putting some substance into this pure assumption during the last twenty years can be seen by comparing the following two statements:
We know remarkably little about evolution in population I after the red giants68 (J. L Greenstein, 1960)
The details of the process by which the red giants evolve into white dwarfs are poorly understood.69 (R. C. Bohlin, 1982)
But when a pure assumption of this kind is repeated again and again, its dubious antecedents are eventually forgotten, and it begins to be accepted as established knowledge. The remarkable way in which the status of this assumption as to the location of the evolutionary path has been elevated by the process of repetition, without any addition to the observational support, can be seen from the following statement from an astronomy textbook, in which the “poorly understood” and purely hypothetical evolutionary course becomes a certainty:
We do not know precisely what happens Ito the red giants] at this point, but we are sure that shortly thereafter the star moves rapidly to the left on the H-R diagram and then downward, fading out slowly in the lingering death of the white dwarf.70
Even in the light of conventional theory, the hypothesis that the stars “move rapidly to the left on the H-R diagram [from the red giant region] and then downward,” meanwhile shedding mass, is untenable. Movement to the left from the red giant region involves an increase in the mass of a Class I star, and either an increase or a constant mass for a member of one of the later classes. The stars in the upper left of the diagram are the most massive of all of the known stars. The mass loss assumed to be taking place during this hypothetical leftward movement is incompatible with the observed mass relationships. Nor is there any explanation as to how this assumed loss of mass could take place. Shklovsky, for instance, concedes that “we simply do not understand exactly how material is ejected from the envelopes of such [red giant] stars.”71
Furthermore, even where matter is actually ejected from a star, this does not necessarily mean that it leaves the system. When the issue is squarely faced, it is apparent that there is no evidence of any significant loss of mass from any star system, other than the stars that explode as supernovae. There are, of course, many types of stars that eject mass, either intermittently or on a nearly continuous basis, but they do not given their ejecta anywhere near enough velocity to reach the gravitational limit and escape from the gravitational control of the star of origin. This ejected matter therefore eventually returns to the star from which it originated.
In this connection, it should be noted that although the relation of the stellar mass to the variables of the CM diagram is different for the different classes of stars, our findings show that it is fixed for any one of these classes. Stars that are following an evolutionary course that involves an increase of mass cannot lose mass and still continue on that course. This not only rules out the theoretical loss of mass by stars such as the red giants which show no evidence of any significant outflow of matter, but also means that the observed ejection of material by stars like the Wolf-Rayets is a cyclical process of the kind discussed in the preceding paragraph. We will encounter this same kind of a cyclical ejection process in a more extensive form in the case of the planetary nebulae, which will be examined in Chapter 11.
The present chapter is the first in this volume that involves a full-scale application of the reciprocal relation between space and time, the most significant consequence of the postulate of a universe composed entirely of motion. Some of the conclusions of the preceding chapters depend in part on this relationship, but the entire content of this chapter rests on the inverse relation between the effects of an expansion into space and those of an expansion into time. The concept of an object becoming more compact (from the spatial standpoint) as it expands will no doubt be a difficult one for many individuals (although for some reason, most seem to be quite comfortable with the fantastic “holes” in space—black holes, white holes, wormholes, etc.—that figure so prominently in present-day cosmological speculations). But the validity of the reciprocal relation between space and time has been demonstrated in many hundreds of applications in the preceding volumes, and it provides the complete and consistent explanation of the white dwarfs that conventional astronomical theory is unable to supply.
The theory of white dwarfs in the universe of motion contains none of the awkward gaps that are so conspicuous in currently accepted astronomical theory. In the context of this new theory both the nature of the white dwarfs and their properties—those properties that are so different from those of the familiar objects of everyday life—are necessary consequences of the event in which these stars originated: the supernova explosion. And these properties define the ultimate fate of these objects. There is no need to assume a stellar “death” for which there is no observational evidence. The destiny of the white dwarf, an eventual return to the main sequence, is implicit in the physical characteristics that make it the kind of a star that it is.
